

|
| Elliott Sound Products | Equalisers |
 Main Index Main Index
 Articles Index Articles Index
|
Contents
Equalisation (EQ) is one of the most contentious areas of hi-fi. For many years, it was expected of any preamplifier that it would have (at the minimum) bass and treble controls. There were untold variations of course, but the general scheme that ended up being used by almost all manufacturers was the 'Baxandall' topology, named after its inventor Peter J Baxandall. This arrangement is used to this day, but for audio production (as opposed to reproduction) the equalisation available is much more complex and comprehensive.
The term 'equalisation' probably came from the requirements of various operators (phone, motion picture, broadcast, etc.) to get their systems back to a flat frequency response - in other words to make it 'equal' to the intended signal.
In reality, equalisation (or simply 'filtering' as it was known in the early years) has been part of recording and PA equipment from the beginning of the technology. Western Electric (which eventually became Bell Labs) described filters (equalisers) for the telephone system to adjust the frequency response and correct high frequency rolloff in the telephone lines. Early 'tone' controls were in evidence not long after the advent of AM radio ('wireless' as it was known at the time). These were typically only able to roll off the high frequencies to make the sound more 'mellow' and reduce extraneous noise.
While audiophiles the world over eschew any form of EQ, at least 99% of the recordings they listen to have already been processed with individual EQ on each channel, as well as overall EQ, compression, limiting, and other 'effects' as may be deemed appropriate by the recording and mastering engineers. However, in this article, I will discuss mainly 'user adjustable' equalisation ('equalization' for North American readers).
Mixing desks for recording and live production provide extensive EQ, and no-one would be silly enough to build a mixer without it. Each channel has a comprehensive tone control network, almost always with at least two bands of parametric equalisation. The term 'parametric' refers to the fact that all the parameters of the circuit are adjustable - frequency, bandwidth (Q) and boost/ cut.
Daniel Flickinger introduced the first parametric equaliser in early 1971 (US Patent 3752928 A). His design used opamps to create filter circuits that were not viable with other techniques. Flickinger's patent ("Amplifier system utilizing regenerative and degenerative feedback to shape the frequency response") shows the circuit topology that was used, and it forms the basis of parametric EQ used to this day.
An earlier form of comprehensive tone control was the graphic equaliser - so-called because the slider pots described a 'graph' of the final frequency response. To be useful, a graphic EQ system needs a lot of separate filters. Octave band graphic EQ systems used 10 slide pots, with one for each octave. More expensive units had 20 sliders (1/2 octave) or 30 sliders (1/3 octave). It was common for these to use ferrite-cored inductors prior to the development of integrated opamps and the invention of the 'gyrator' circuit. A gyrator uses an opamp, resistors and a capacitor to simulate an inductor (hence the generic name 'simulated inductor').
It's often been stated that "tone controls are provided so the user can mess up the sound". In many cases this is certainly true, but it has to be considered that the end-user is perfectly entitled to mess up the sound if s/he wants to do so. This article is not about ultimate sound quality, but the various types of equaliser that are available, and how they work.
It's also worth your while to browse the various circuits from the ESP projects list. There are quite a few different types of equaliser described, ranging from simple bass and treble controls through to quasi-parametric designs, graphic equalisers and fixed EQ systems for low frequency response extension for loudspeakers and subwoofers.
 | Note that all the circuits shown below rely on a low or very low impedance source. This can be an opamp (best), transistor emitter follower (ok) or a valve cathode follower (worst), depending on the other circuitry used. So, although input buffers are not shown they are essential in all cases. This still applies where the input uses an inverting opamp stage, because the insertion loss of the circuit depends on a low source impedance. |
The circuits below are not for construction (although you can do so if you wish, but don't expect assistance). Because they are not projects, none has been built as shown, and although all have been simulated no other tests have been done. Likewise, there's been no attempt to optimise the circuits for any particular task, so they may not be found suitable as described. I will respond to queries about projects, but I will not provide assistance to anyone to build any of the circuits shown here.
The most common fixed EQ circuit is that used for RIAA vinyl phono playback from magnetic pickups. Although there is vast number of different topologies, the end result is pretty much the same. RIAA playback EQ provides bass boost and treble cut to match the disc cutting process. This (by design) cuts the bass response so the grooves aren't so wide as to cut into adjacent grooves, and boosts the treble as a form of pre-emphasis. Upon playback, the treble cut reduces the disc's surface noise sufficiently to produce a fairly quiet end result.
Other common fixed equalisers are or were used with recording tape, FM broadcast, long phone lines used for radio or television distribution and a multitude of other systems. Pre-emphasis (treble boost) and de-emphasis (complementary treble cut) increase the apparent signal to noise ratio (SNR) and these have been used for many years. Pre-emphasis is used in FM broadcasts, and the receivers have a complementary de-emphasis circuit that gives an overall flat response.
Fixed equalisers can also be used to allow a loudspeaker to achieve (or attempt) 'full range' from single loudspeaker drivers. One of the best known is probably the Bose 901, which uses 9 × 100mm (4") drivers and has a 'line level' equaliser that supposedly produces flat response (although it also has some tone control available). Many subwoofers use a fixed equaliser to get as low as possible even in a small enclosure.
Modern systems using DSP (digital signal processing) may also qualify as 'fixed' EQ, because after the setup process is complete there is usually no facility to adjust the relative levels. There's also a movement to apply EQ to 'correct' the speakers for the room, but this is a flawed concept for the most part, other than for frequencies below ~100Hz or so. In a nutshell, you cannot equalise a room, because most of the problems are caused by anomalies in time, and you cannot correct time with amplitude.
Fixed EQ is also used in smartphones, tablets and laptops, usually both for the inbuilt microphone and speakers. The amount and type of EQ depends on the manufacturer, but it's safe to say that it will usually be done using DSP. Some may allow applications to disable the microphone EQ (and compression) for wider frequency and dynamic range. Another form of fixed EQ is a notch filter, and these can be extremely narrow and used to remove an unwanted frequency. An example is the 19kHz notch filter used in FM receivers to suppress the 19kHz pilot tone that's used for stereo broadcasts. Notch filters can also be used to remove 50/60Hz hum from a signal without greatly affecting nearby frequencies.
The primary purpose of this article is to describe user adjustable controls, not fixed EQ systems. Therefore I shall not delve into the realm of fixed equalisers other than in passing.
The early forms of boost/ cut tone control circuits were passive, and had a significant insertion loss. Because there was no active circuitry in the circuit itself, in order to be able to boost the bass or treble, the overall signal was attenuated. Simple filter circuits allowed the end user to independently set the bass and treble controls to obtain a sound that was pleasing to the listener. Accuracy was never a consideration, and the setting used was purely subjective.
Probably one of the earliest use of equalisers for audio was to try to get decent (and intelligible) sound from early movie soundtracks [ 3 ]. It's not known if there were any equalisers used for radio broadcast, but I'd be surprised if at least some form of (perhaps fixed) filtering wasn't applied to compensate for deficiencies in the transmitter modulators and other parts of the transmission chain. There was definitely a requirement to limit the bandwidth, because AM transmission cannot be allowed to be full frequency range due to the problem of potential adjacent station interference. These don't qualify as tone controls though, because they had fixed frequency response. The same applies to 'equalisers' used to correct phone-line transmissions.
The top-cut style of tone control was standard on most mantel radios and even record players up until the late 1960s. In the valve era, it wasn't possible to include 'proper' tone controls in budget equipment because valves were expensive, and at least one triode was needed to bring the signal back to normal level. Although there were many 'high end' hi-fi systems and construction projects published in Wireless World (UK, now Electronics World), R,TV&H in Australia (Radio, Television & Hobbies) and Practical Electronics (US) and many other magazines, only the more affluent enthusiasts could afford the off-the-shelf equipment that had the latest and greatest tone controls (and other specifications to match).
There was a period where the best equipment available was expected to have tone controls. The Quad 22 preamp was an example, and that had quite sophisticated controls, featuring bass and treble as expected, but also having a switchable low pass filter (5kHz, 7kHz and 10kHz) to help reduce noise from the signal source. At that time (1950s to 1960s and beyond), nearly all preamps had tone controls, and many innovative new topologies were developed to provide more control over how the controls functioned. Some allowed for quite radical amounts of boost and cut. Up to ±20dB wasn't unheard of, but most were limited to a more sensible ±12dB or so.
When graphic equalisers were first introduced to home hi-fi systems they were usually very basic. Some had as few as five bands (2 octave range), and although quite limited gave the home listener plenty of scope to mess up the sound. However, if the end result made the owner happy then that's all that really mattered. With most systems today, the inclusion of DSP (digital signal processing) allows the user to select any number of 'effects' that can ruin everything with far greater ability than anything that has come before.
Most simple tone control circuits use the simplest type of filter - resistance/ capacitance (RC) networks that provide a theoretical maximum slope of 6dB/ octave. Those using capacitors and inductors (real or simulated) can achieve far greater slopes, but are configured as band-pass or band-stop (depending on the pot position). Graphic equalisers come in two major formats too, with the most common types providing a variable Q (bandwidth) depending on the amount of boost and cut. The other type is 'constant Q', patented by Ken Gundry of Dolby Laboratories and further developed by Rane. These have a (more or less) constant bandwidth regardless of the amount of boost or cut.
The Langevin Model EQ-251A was the first equaliser to use slide controls, but in this case they were slide switches, not pots as we expect today. It used only passive sections, and each filter had switchable frequencies and used a 15-position slide switch to adjust cut or boost. The first true graphic equaliser was the type 7080 developed by Art Davis's Cinema Engineering. It featured 6 bands with a boost and cut range of 8dB. It used a slide switch to adjust each band in 1 dB steps. Davis's second graphic equaliser was the Altec Lansing Model 9062A EQ. In 1967 Davis developed the first 1/3 octave variable notch filter set, the Altec-Lansing 'Acousta-Voice' system.
It's important to understand that there is a vast difference between the tone controls that may be used on a hi-fi or mixing console and those used in guitar or other musical instrument amps. In hi-fi or mixers, it is essential that a flat response is available, simply by setting the boost/ cut controls to centre. The circuit then has no effect on the response, so what goes in comes out without change. With musical instrument amps, the situation is very different. The tone controls work in conjunction with the instrument, pickups and the loudspeakers, and the overall effect is to provide a wide range of 'tones' through the speaker that are pleasing to the musician.
For example, a guitar amp is not intended to reproduce sound, it's intended to create (produce) sound. The amplifier and speaker system form part of the instrument - any one without the other is pretty much useless. Try playing a well liked recording through a guitar amp - you will never get it to sound right. Much the same happens if a guitar is played through a hi-fi system. Even if it has tone controls, it will be difficult or impossible to get 'the sound' that a guitarist is used to hearing, and you'll probably end up with blown tweeters to add injury to insult (as it were).
Early guitar amplifiers often had no more than a 'top cut' tone control, but users wanted more. The 'tone stack' as it's generally known now was developed fairly early, but despite much searching I was unable to find out who designed the first version. The guitar amp style tone stack is only capable of providing bass and treble boost (which equates to a midrange cut). The midrange control only lifts the average level across the frequency range, and is deliberately limited so it doesn't render the bass and treble controls inoperative. In most designs, there is no setting that has a flat frequency response - all you can do is vary the amount of bass and treble boost. These circuits are always passive, and have an insertion loss of 20dB or more. Insertion loss simply refers to the amount of signal you get at the output vs. the input, with the controls set to flat or the closest to 'flat' that the circuit can provide.
A few designers over the years have used Baxandall (feedback) tone controls in guitar amps (often as magazine projects), and most qualify as bloody awful at best, unusable at worst. This isn't to say that they can't be used, but in general guitarists will not be at all happy with the end result. To anyone who has designed a guitar amp or two (or three, or ...) this comes as no surprise. Music production and reproduction are very different, and cannot be considered equal in any way. While electric guitar can be especially hard to get right, bass guitar and acoustic guitar with magnetic or piezo pickups can also be very demanding.
The simplest, most basic and least useful tone control simply provides bass and/or treble cut. These are easily created and were very common in many earlier wireless sets. Bass cut wasn't so common, but nearly all mantel radios from the 1940s onwards featured a 'tone control', which was nothing more than a variable treble cut. By varying a pot, the high frequency response could be rolled off to allow the user to obtain a 'mellow' sound that had a very restricted top-end. Even from an early age, I found that setting the tone control to the position that gave the most treble (such as it was with an AM mantel radio or similar) was far more satisfying than the muffled sound that my parents seemed to prefer.
The general principle is shown below. No boost was possible for bass or treble, simply because early radios and record players barely had enough gain to reach full volume even without any tone control, so reducing the gain to allow boost for separate bass or treble controls wasn't an option. Gain was expensive, because it required another valve stage. The important part here is that if you want to be able to boost bass or treble with a passive network, the entire signal has to be reduced so the filters can be adjusted to provide an apparent boost. Simple bass and treble cut controls are shown below, as these are the most basic of all.
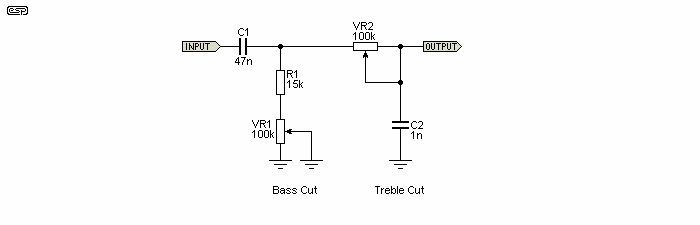
These controls have the minimum possible effect on the rest of the signal, so they could be added without any gain penalty. This meant that an additional valve or transistor wasn't needed, so the cost of including them wasn't great. A couple of potentiometers, knobs, resistors and capacitors was all that was needed. With both controls set for maximum cut, the effect was to provide a signal that was all midrange - no bass, no treble, only the mid frequencies. However, if the two are combined there will be some interaction.
Note that as the controls are adjusted, they can only cut - there is no facility to boost the signal at any frequency. The treble cut control reduces the level by 6dB/octave from a turnover frequency determined by the pot position and the bass cut control does the same. Treble control can also use a variable capacitor, but that was never appropriate because of the physical size of a variable capacitor with enough capacitance to be useful. It can be done easily with a capacitance multiplier, but these were never used in the valve era and remained uncommon until opamps became readily available. With the values shown, the -3dB frequency response with both controls set for maximum cut is from 177Hz to 2kHz. With the pots set for minimum cut the response is essentially flat from 30Hz to 20kHz. The circuit must be followed by a high impedance stage and fed from a low impedance.
If you need to apply boost at any frequency, you need to accept a loss that's slightly greater than the boost allowed or incorporate a gain stage. This can be a valve, transistor, FET or opamp, depending on the era of the design. Early cut/boost tone controls were passive and could introduce a loss of as much as 20dB with the pots centred (flat response). This loss had to be made up by adding a gain stage.
The general scheme seen below is often referred to as a 'James' EQ, so called because it was first published by E.J. James [ 1 ]. You may also see it referred to as a 'passive Baxandall', but that's not correct. The design published by Peter Baxandall is active, and uses feedback to get symmetrical boost and cut. The Baxandall tone control requires an inverting amplifier stage with low output impedance to drive the filter circuits. The James circuit requires a low source impedance and high impedance load, or performance will suffer.
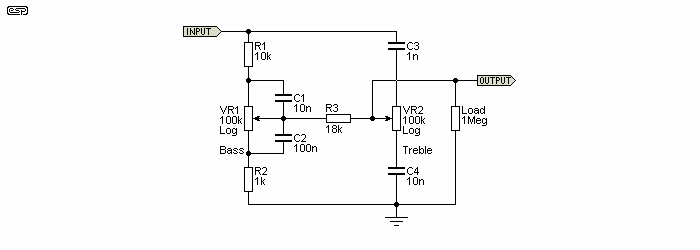
There are countless variations on this basic circuit. As shown, it's one of the more common arrangements and allows a nominal cut and boost of around +18dB and -20dB (it's not perfectly symmetrical). The bass and treble turnover (±3dB) frequencies are changed by using different capacitor values. Smaller caps work at higher frequencies. The bass section can use one capacitor (in parallel with VR1) or two as shown. The treble section may also use two caps as shown, vs. a single cap in series with the wiper of the treble pot.
There is a slight difference between the circuit variations. Tone control circuits must be driven by a low output impedance (cathode or emitter follower), and there is some interaction between the controls with most passive versions. A true flat position is difficult to achieve with the Figure 2 controls, and a frequency deviation of up to ±2dB is not uncommon. Note that the pots are logarithmic - linear pots do not work, but log tapers are rarely good enough to ensure front panel calibration for flat response. Insertion loss is about 20dB. The following stage must have a high impedance input, and direct coupling to the grid of the following valve (with no additional grid resistor) was not uncommon. A gain of 10 is needed to restore the level with the controls set for a nominally flat response.
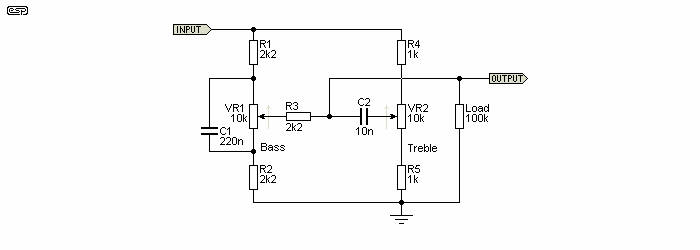
The above shows a very simple EQ circuit that I devised a great many years ago for simple stage mixers and 'pre-mixers'. The idea was to provide some control, but not so much that it would get inexperienced users into trouble. The basic scheme is superficially the same as that shown in Figure 2, but the components are the same value for the 'top' and 'bottom' parts of the circuit (compare this with Figure 2). The insertion loss is small (6dB with the controls centred), and the maximum boost is limited to a little under 6dB. There is more cut available, but that only becomes apparent with the control(s) set for minimum bass or treble cut.
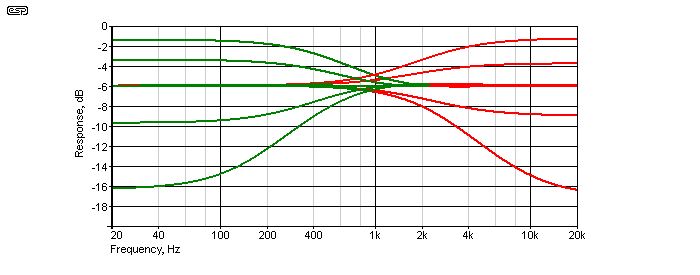
Response of the bass pot is shown in green, and treble in red. The pots are linear, and graphs are shown at 25% increments. Unlike the version shown in Figure 2, when the pots are centred the response is completely flat, with almost no deviation at all. There is a small deviation that can be measured, but it's below audibility (about 0.3dB with a 100k load, or 0.03dB if loaded with 1 megohm).
Interestingly, the Figure 3 circuit is almost exactly what you'd expect to see used with an inverting gain stage in a Baxandall control circuit [ 2 ]. The same values used with an inverting gain stage give perfectly symmetrical boost and cut, with a maximum of ±15dB with the values shown. This type of control is shown next, and was very common in home hi-fi systems and mixing consoles. The circuit is seen below, using the exact same component values as shown in Figure 3, but with the addition of an opamp gain stage.
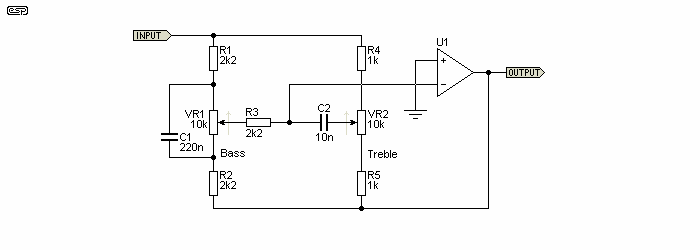
This type of circuit is possibly the most popular of all time. Some manufacturers have provided switchable capacitors so the response can be tailored to the user's preferences. There are variations with a midrange control, which is achieved by adding a third pot that has a cap in parallel (like the bass control) and another (smaller) cap in series with the wiper (like the treble control). When a midrange control is included, it's almost always fixed - to make it variable requires switched capacitors.
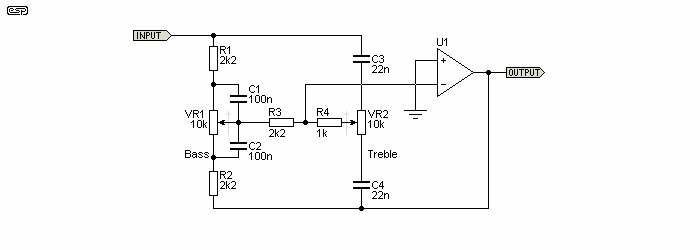
You will often see the version shown in Figure 5B, using a pair of caps for both bass and treble. The response is similar to the version shown in Figure 5, but there are some subtle differences. There's a little more 'disturbance' in the midrange with the 5B circuit, and it has a little more boost for both bass (~1dB at 28Hz) and treble (~3dB at 20kHz). Cut is (almost) identical, but the frequencies are shifted slightly because the caps aren't exactly half/ double those shown in Figure 5. The alternative 5B circuit uses twice as many capacitors, and IMO is inferior to the Figure 5 circuit. Essentially it's a symmetrical version of the Figure 2 network, enclosed in a feedback loop.
The generic term for equalisers with the type of response provided by James and Baxandall tone controls is 'shelving EQ', because the bass or treble is boosted by a set amount, but then returns to being almost flat above or below a frequency that's determined by the setting of the control pot. You can see this in Figure 4, the boost and cut level out below 200Hz and above 4kHz. Because the Figure 3 circuit is passive and has no feedback, at maximum cut the bass doesn't level out until about 60Hz, and the treble doesn't really level out at all. Once feedback is applied, this changes as shown in Figure 6.
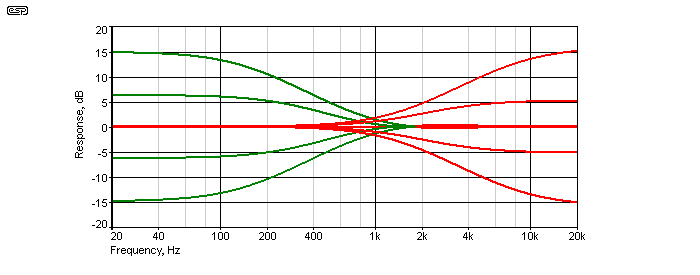
Colours and pot increments are the same as used for Figure 4. You will notice that boost and cut are now (almost) perfectly symmetrical. Remember that these plots used the exact same tone filters as shown in Figure 3, and the only difference is the addition of feedback.
The full performance and symmetry of Baxandall circuits was difficult to realise with valve circuitry, because getting a very low output impedance from the drive and feedback stages was extremely difficult. As is common with all valve circuits, the tone control networks were high impedance, using 100k or higher for pots, and with other components scaled to suit. It became easier when transistors were used, and was virtually automatic when opamps were used as the source and amplifying devices. Perhaps some of the nostalgia for valve circuitry was the rather 'sloppy' response obtained due to relatively high impedances. This can be restored (why?) by adding resistors in series with opamp outputs.
It's to be expected that some people will insist on passive controls, because they imagine that applying feedback somehow ruins the sound. This is complete nonsense of course, and there seems little point in using a vastly inferior tone control system that has no real flat setting just to avoid the 'evil' of feedback. If this approach is taken, only the Figure 3 circuit is really suitable, because a flat setting is possible and dubious (at best) log pots are not needed.
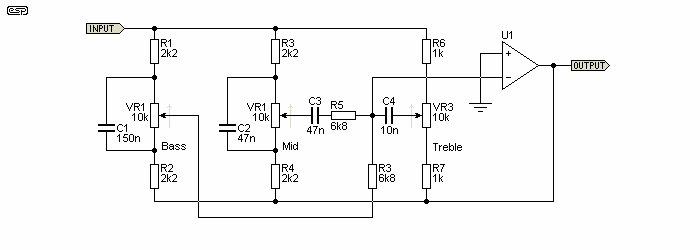
In the interests of completeness, the above shows the general arrangement used to add a midrange control to a Baxandall network. The Q is low (about 0.5) and you can't adjust the frequency easily, but it does add some extra functionality that might be useful for a musical instrument amp. While you may see it added in many circuits on the Net, it's of somewhat dubious value. Because it's not easily adjusted for frequency (C2 and C3 can be changed, optionally with switches), due to the low Q most users are likely to find it doesn't really do what they need. To increase the 'midrange' frequency, reduce the value of C2 and C3 and vice versa. The values will normally be the same, but that's not essential.
Calculating the component values to set specific frequencies is possible, but it's far from precise. The controls are always somewhat interactive, and because they use pots the resistance is variable. Texas Instruments has shown some formulae in various datasheets, and while they work they aren't particularly accurate and are simplifications. For the most part, it's far easier to use the data from an existing design and just scale the capacitor values. If the capacitance is doubled, the frequency is halved and vice versa. Intermediate values can be estimated quite well. For example, if the capacitance is increased/ reduced by a factor of 1.5, the frequency is changed by the same fraction.
These filters all have low Q (generally less than 0.5), and the frequency for ±3dB of boost/ cut is not fixed. It varies with the amount of boost/ cut, so attempting to create a formula is more trouble than it's worth. If you use a simulator you'll be able to get accurate results, but ultimately it's about the sound. If you get the sound you want then that's all that matters. This is particularly true for guitar (and other musical instrument) amps, but it also applies for hi-fi.
While the basic shelving filters described above are fine for controlling bass and treble, to affect the midrange or a troublesome frequency anywhere in the audio band isn't possible. In many cases bass and treble controls don't even work for bass and treble. For example, if you want to get a 'fat' kick drum sound you might add some bass, but you don't want or need to keep boosting all the way down to a few Hertz. Look at Figure 6 - if you have 10dB of boost at 70Hz, you have slightly more than that at 40Hz and it's still there at 20Hz. A peaking filter can be tuned to 70Hz (for example) to give a satisfying 'thump' from the kick drum, but the level returns to normal (towards 0dB gain) as the frequency increases or decreases.
Graphic equalisers have a series of bandpass filters, with each frequency band controlled by a slide-pot. Each frequency can be cut or boosted, and uninformed fiddling can cause problems. There was a brief period where stereo graphic EQ was considered a 'must' for what's probably better known as 'low-end hi-fi' - comparatively cheap systems that made up for the lack of overall quality by including extras that made the buyer believe s/he was getting a good deal.
This general form of equaliser was developed in the early 1970s, and inductors were used as part of the frequency selective networks. Inductors are comparatively large, require many turns of wire and a magnetic core (steel laminations or ferrite). They are expensive to make, and nearby magnetic fields can induce hum into the windings.
Graphic EQ was therefore expensive and quite bulky until the invention of the gyrator (a 'simulated' inductor, using an opamp to invert the action of a capacitor). Although the gyrator was proposed in 1948 (by Bernard Tellegen, a Dutch engineer who also invented the pentode valve), practical realisation wasn't possible until opamps became readily available. Very basic gyrators can be made using only a transistor, but their performance is sub-standard. I don't know of anyone who has tried to make a gyrator using valves because it would not be sensible. The active element of a gyrator is a non-inverting unity gain buffer, which should have high input impedance and low output impedance.
Gyrators allowed designers to create large numbers of 'inductors' very cheaply compared to true inductors, and gyrators are unaffected by magnetic fields so induced hum was no longer a major problem. The general form of a graphic equaliser is shown below, but using inductors for clarity. It doesn't matter if the inductor is 'real' or simulated, it has exactly the same effect. Note that the value of the resistor (R2, R3, etc.) is often the winding resistance of the inductor, and/or an external resistor used to ensure that the series resistance of each tuned circuit is identical. In the following drawing, only the first 5 octave band filters are included. The remainder follow the standard octave frequencies. Industry standard frequencies for the three most common equalisers are ...
| 31 | 63 | 125 | 250 | 500 | 1k0 | 2k0 | 4k0 | 8k0 | 16k |
| 31 | 44 | 63 | 87 | 125 | 175 | 250 | 350 | 500 | 700 | 1k0 | 1k4 | 2k0 | 2k8 | 4k0 | 5k6 | 8k0 | 11k | 16k | 20k |
| 25 | 31 | 40 | 50 | 63 | 80 | 100 | 125 | 160 | 200 | 250 | 315 | 400 | 500 | 630 | 800 | 1k0 | 1k2 | 1k6 | 2k0 | 2k5 | 3k2 | 4k0 | 5k0 | 6k3 | 8k0 | 10k | 12k | 16k | 20k |
The frequencies shown above are pretty much agreed upon worldwide, and have been adopted by all manufacturers making graphic equalisers. The 1/2 octave and 1/3 octave frequencies are often extended above and below those shown, and may include 20Hz and/or 25Hz, as well as 20kHz. The drawing below shows ideal values rather than those readily available, purely for convenience. The Q of each filter is about 2, extreme accuracy is not really possible and fortunately isn't necessary. The circuit below must be driven from a low impedance. Normally, there would be a unity gain buffer to drive the input. It isn't shown but must be included unless the previous stage is an opamp or other very low impedance source.
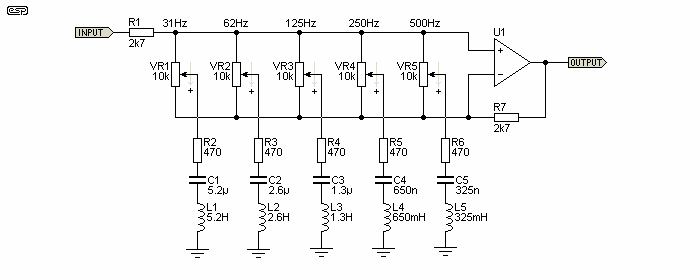
Without the frequency selective networks (C1, L1, etc.), the pot sliders simply vary the gain of the circuit and unity gain is achieved when the slider(s) are centred. When the pot wiper is close to the input (+ve input of U1), the incoming signal is attenuated (cut), and at the opposite end (shown with a + sign) the opamp has gain (boost). When each pot connects to a tuned circuit, only the frequencies passed by the tuned circuit are affected. In circuits developed after ca. 1970 or so, the inductor is replaced with a 'simulated inductor' - a gyrator.
The tuned circuit filters have a minimum impedance at a particular frequency as shown, so the pot affects only those frequencies passed by the filter. A series resonant circuit has a minimum impedance at the resonant frequency, and this forms the basis of most simple graphic equalisers. The scheme shown above gives an equaliser whose actual Q (as opposed to the theoretical value) varies with the slider setting. At low boost or cut settings the bandwidth is much wider than expected.
One thing that is fairly difficult to find explained in simple terms is just how to determine the inductance and capacitance needed for a specific Q. The values depend on the load (series) resistance, which in the above circuit is 470 ohms. The impedance (X) of the cap and inductor must be scaled to the load resistance (RL), and the following formulae apply ...
X = RL × Q
C = 1 / ( 2π × X × f )
L = X / ( 2π × f )
The Q (which determines the bandwidth) of each filter depends on the number of sliders used (10, 20 or 30). A 1/3 octave graphic EQ needs higher Q filters than a 1 octave band type. Q is defined as the centre frequency divided by the bandwidth, and a 1 octave filter requires a Q of 2. A 1/3 octave EQ system needs filters with a Q of 4.31 (4 is close enough for an equaliser). You may well ask why the Q isn't constant, and the answer is quite simple.
When the pot is near the centre position, the load on the tuned circuit is no longer 470 ohms, it's 470 ohms plus the equivalent resistance of the pot and the feed resistors (2.7k as shown). As the pot position varies, so does the Q, and therefore the bandwidth changes as well. This type of circuit cannot provide a constant loading on the tuned circuit, so cannot provide a constant Q. The effect can be reduced slightly by using lower value pots, but that increases the noise gain of the opamp, so the circuit will become noisy even with a low noise opamp. The opamp inputs are not virtual earth types, and both have a relatively high impedance.
A constant-Q graphic equaliser suitable for subwoofer equalisation is described in Project 75, and this arrangement was first published by Bob Thurmond [ 5 ] and is shown next. Commercial units were pioneered by Rane [ 6 ], but using a different circuit.

It's important to understand how this circuit differs from the previous version. The most obvious difference is that the opamp inputs are both virtual earth (close to zero impedance), and the band pass filters are not RLC types as shown above. You may see a variety of different active bandpass filters used. Typical types are multiple feedback, twin or bridged tee or even state-variable. It is possible to use RLC filters (resistance, inductance, capacitance), and gyrator based filters can be used with some extra circuitry, but the other filter types remain a simpler and better choice.
You can see that the pots control the output from each band-pass filter (BPF), and the multiple outputs are summed along with the input signal at the input to U1 (signal cancellation or cut) or U2 (signal augmentation or boost), depending on the pot position. When the pot is centred, the signal to U1 and U2 is identical, so it cancels and there's no boost or cut for that frequency. The Q remains constant because it's only the output from the appropriate BPF and the load doesn't change.
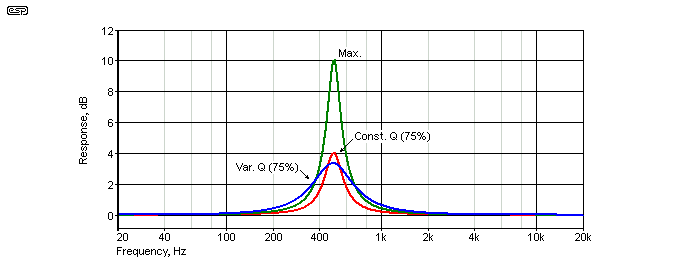
Above you see the response of two equalisers, one configured as a traditional graphic EQ and the other configured for constant Q. Assuming equal bandwidth for each, both will have the same response at maximum boost or cut, but the situation is quite different at any setting below maximum. The setting for constant Q vs. variable Q is shown for a pot setting of 75% (50% boost). Cut response is the same (but results in a dip of course).
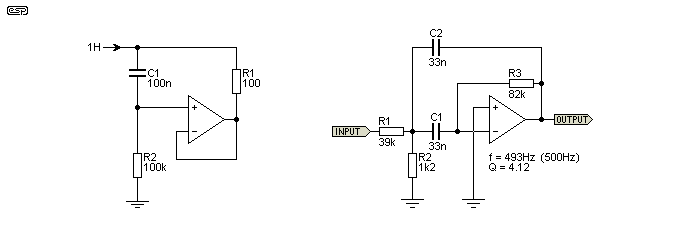
The general topology of a gyrator and band-pass filter are shown above. The effective inductance of a gyrator is simply the product of the three components (R1, R2 and C1). When the three are multiplied together, the answer is the inductance in Henrys. Equivalent winding resistance is the value of R1. Gyrators are covered in greater detail in the article Active Filters Using Gyrators - Characteristics, and Examples. The multiple feedback bandpass filter is a simple and fairly straightforward design, although calculating the values can be very irksome. They are described in detail in Project 63, and there's even a calculator program available that you can use to work out the component values for you. The multiple feedback filter is not easily tuned, and when variable frequency is needed the choice is between the various bandpass filters covered in the section about parametric equalisers.
While a graphic EQ is certainly a very flexible way to control the frequency response, they need a large number of slide pots and therefore take up a lot of room. This makes their use on mixing consoles (for example) limited to perhaps a couple of graphic EQs for the main or 'FOH' (front of house) outputs. There simply isn't enough space on each channel strip to include one for each channel, and simple bass and treble controls are too limited.
There is one other type of graphic equaliser that deserves at least a mention. As far as I'm aware, only a (small) few manufacturer ever produced them, one being IRP (Industrial Research Products). The form of filter uses an analogue delay line, typically made up with a number of all-pass filters (phase shift networks). The delayed outputs are then fed to a very complex resistor matrix, and finally to summing amplifiers for each band.
These are superficially simple, but in practice are very complex. A 31 band (1/3 octave) version needs well over 100 opamps, and a resistor matrix using hundreds of resistors of different values. Even if I had a complete circuit, it would be so large as to be impractical for publication (and I'd need permission to do so). I don't have much useful information on these, but the technique is certainly interesting, based on the small amount of information I have available.
To get the benefits of EQ that can be tailored to the exact needs that doesn't occupy too much space on a channel strip requires a parametric equaliser, discussed below.
Simple bass and treble controls can benefit from having adjustable frequencies. It's no longer possible to use the Baxandall topology, so it's done using various other techniques. The easiest is to use the same basic arrangement as used in common graphic equalisers. There have been many schemes used, but most use a variable frequency high and low pass filter in a feedback network. A few (including some that I designed) use an opamp to create a variable capacitance (a capacitance multiplier), and others have used a variety of circuits. It would be silly to try to include them all, so only two variants are shown.
The first is fairly conventional, and there are quite a few references to very similar circuits on the Net. The circuit consists of two inverting gain stages and two unity gain buffers. The latter isolate the boost and cut controls from the frequency networks, and are essential to prevent unwanted interactions. VR1 changes the bass frequency from 200Hz to 740Hz at the ±3dB point. VR2 does the same for the treble, from 460Hz to 1.4kHz, again at the ±3dB frequencies with full boost or cut.

The above circuit works well and is not critical, and component values can be changed to increase the frequency range or provide more (or less) boost and cut. As a general purpose tone control, it's far more flexible than the standard Baxandall circuit, but gives almost identical results for any given frequency setting. A better solution uses a variable gyrator for the low frequencies and a variable capacitance multiplier for high frequencies. This has the advantage that the bass control can be switched from shelving to peaking, and additional sections can also be added.

The gain and boost/cut circuitry is identical to that used for a conventional graphic EQ, and is easily expanded as described in the Project 28 page. As a parametric equaliser it's not wonderful, but it's still surprisingly effective. If you only want variable frequency bass and treble controls it's better than the circuit shown in Figure 12 because you get the option of shelving or peaking for the bass control. As noted above this can be especially useful for percussion (kick drum, toms and kettle drums for example). The circuit uses three unity gain buffers and one gain stage. The input buffer is not needed if the source has a low output impedance, such as from another opamp in the circuit.
Be aware that the variable capacitance multiplier (U3) can be temperamental. On occasion, it may not settle properly to normal quiescent conditions (output at zero DC voltage), and it might need to be powered off and on again before it settles down. I've been unable to replicate this on the workbench, so it seems that the circuit knows when test equipment is nearby  . Mostly it works perfectly - I have one in an equaliser I use for my workshop system that's never missed a beat in over 20 years.
. Mostly it works perfectly - I have one in an equaliser I use for my workshop system that's never missed a beat in over 20 years.
In shelving mode, the circuit works almost identically to that shown in Figure 12. The range of each frequency control can be changed by using a higher (or lower) value pot, and the frequencies are changed by replacing C1, C2 and/or C3 with values that provide the desired ranges. For the peaking filter section (C1 in circuit), the ratio of C1 and C2 determines the resonant circuit Q (C2 determines the inductance of the gyrator). Normally there is an optimum ratio (typically around 10:1) for C1 and C2, but because the inductance is variable vis VR3, the optimum ratio can't be maintained.
There is one thing that the Figure 13 circuit does that is not especially desirable, When in peaking mode, the Q changes depending on the setting of VR3. At very low frequencies the Q is higher than at higher frequencies. This variable Q is either a benefit or a curse, depending on what you want to do. With the values shown, the Q ranges from 9.5 to 2.0 (at maximum boost or cut, and at 35Hz and 150Hz respectively). At settings below the maximum cut or boost the Q is reduced. It's normal for this type of equaliser, and if you need a circuit that has consistent Q you need a proper parametric EQ as described next.
The most flexible EQ that occupies the least space is a parametric equaliser. Provided the bass can be switched from shelving to peaking mode (and many can), you can insert a peak or dip anywhere you like to get the sound you want. Parametric EQ ranges from simple fixed bandwidth types (such as the one shown in Project 28) through to fully variable 'true' parametric equalisers based on state-variable filters. Simple versions like the P28 circuit provide no control over the bandwidth (Q), but are nonetheless very flexible and can perform most 'sound-shaping' EQ tasks very well. Variable Q is needed if you happen to have a requirement to notch out a particular troublesome frequency. High Q (narrow bandwidth) peaks are rarely needed and if used can create problems with the final mix.
It's almost unheard of to use parametric EQ for a home system. These equalisers are not easy to drive, and should only be used by those who understand how they work and what they do. If adjusted incorrectly it's quite possible for an inexperienced user to not just mess up the sound, but it may be possible to kill tweeters if a substantial boost is applied close to the crossover frequency, so the tweeter receives too much energy at its lowest recommended frequency. Home systems aren't just operated by adults, and kids like to experiment! Hi-fi manufacturers assume (not unwisely) that the average user would be confused by all the options provided, and most 'high-end' equipment offers no form of tone control at all.
As with graphic equalisers, a parametric EQ can be configured for variable or constant Q. Each requires a different approach to the circuit. There are countless variations for parametric equalisers, but the best all-round filter network is the state-variable topology. This is a relatively complex circuit, but has the advantage of being easily adjusted both for frequency and Q. Demands on the opamps are fairly modest and comparatively cheap opamps can perform well.
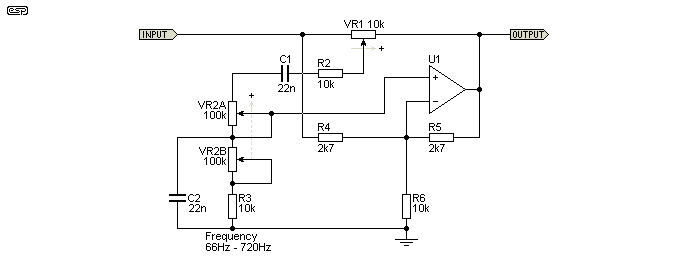
A simpler version uses a Wien bridge as the variable frequency element. These really qualify as 'quasi parametric' EQ, because the Q is fairly low (around 1.3) and can't be changed. However, they are well behaved and easily tuned. A variable frequency stage needs only one opamp in its simplest form, and the tuning network is completely passive. It might seem unlikely that this would be useful, but the Q is actually much greater than a 3-band Baxandall tone control (which only manages a Q of about 0.5), and it can be tuned with a dual-gang pot. This network is not suitable where high levels of boost or cut are needed, as the circuit will oscillate if the gain is too high (set by R6). Without R6 the maximum boost and cut is 9dB and with the values given the maximum is 12dB. The performance can be improved a little by adding a buffer between the pot and the Wien bridge network, but in general the benefit does not outweigh the added expense. There's a lot more info on this topology in Project 150. The Wien bridge network consists of VR2 (A & B), R2, R3 and C1, C2.
Most 'true' parametric equalisers use a state-variable filter (see State-Variable Filters for a detailed analysis). Although comparatively complex, the state variable filter gives independent control of Q and frequency. There are many variations on the scheme, but the end result is fairly similar. In the following drawing, the control section is identical to that shown in Figure 14, and the filter is simply changed from a Wien bridge to a state-variable.
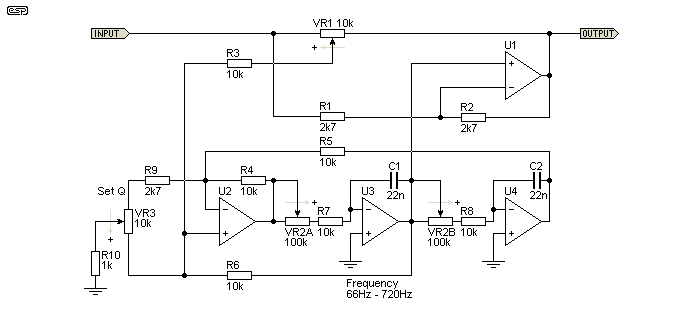
VR3 controls the filter Q without affecting the gain, and VR2(A & B) controls the frequency. With the values given, the frequency range is exactly the same as the EQ in Figure 14, because the values that determine the frequency are the same. The Q can be varied between 5.3 down to 0.5 which gives a very wide control range. Note that VR1 (cut/ boost) operates opposite to the way it does with the Wien bridge circuit. As shown, boost and cut are limited to 9.5dB, but this can be extended by adding a resistor from the inverting input of U1 to earth. If a 2.7k resistor is added, boost and cut are increased to 12dB.
Parametric Equalisers come in multiple types, and usually include variable frequency bass and treble controls, along with one, two or sometimes three bands of true parametric. Frequency ranges usually overlap, and care is needed to ensure that boost isn't used with two sections tuned to the same frequency. There is always a chance that the equaliser will clip, or the output at one frequency will be so high as to place horn compression drivers (in particular) at risk of damage.
When very high Q is used, it's generally only needed to cut a troublesome frequency. High Q boost is rarely needed other than for a special effect. Because the parametric EQ is so flexible, it takes some time to get used to using it properly. Most DAW (digital audio workstation) software includes digital parametric EQ, and there are some on-line tutorials [ 7 ] that explain how the EQ should be used. One of the general tenets of parametric EQ is to "cut narrow, boost wide", referring to the Q or bandwidth of the filter(s). A high Q notch can be very useful, but boost should normally be low Q and kept to the minimum whenever possible. A high-Q boost will almost certainly cause feedback in a live sound system, and can easily damage high frequency drivers.
The 'tone stack' as it is commonly known is only suitable for guitar or other musical instrument amps. It's very difficult to know where it came from (opinions abound, but proof is hard to come by), but tone stacks are used by most guitar amp manufacturers almost exclusively. The arrangement is quite different from a traditional passive control network, and the control pots are wired in series to form a 'stack' (hence the name). They are very economical, and use the minimum possible number of parts, but the controls are usually highly interactive and there is almost always a significant midrange 'scoop' (essentially a broad notch).
Since electric guitars in particular usually have a quite prominent midrange with little bass or extended treble, the midrange scoop makes up for that by boosting the bass and treble and suppressing the midrange. Varying the bass and treble controls shifts the notch or 'scoop' centre frequency and its depth. Where a 'midrange' control is included, the closest to flat response is obtained with bass and treble at zero, and midrange at maximum. A true flat response is usually impossible though. The controls are used to get a guitar sound that suits the player, and the tone controls (as well as the speaker, cabinet and power amp) are used to create sound. The amp has to be considered as part of the instrument, as most guitarists will choose an amp based on the overall sound they can get from the pairing of guitar and amplifier, and linked to their playing style.
There are very wide differences between tone stacks, not only between different manufacturers but even between different models from the same maker. Most are high impedance and are designed for use with valve stages. For best performance they should be driven from a cathode follower, but in some cases even that is abandoned. While guitarists will think that the tone stack in their favourite amp is a work of art, they are really very basic and usually don't work well with sources other than guitar. From a manufacturing perspective, these are the cheapest possible options for tone control, but it just so happens that the characteristics are pretty close to ideal for the purpose. Only a few designers have strayed, and those who were silly enough to try using Baxandall (active) tone controls have never been well received. An Australian magazine once published a guitar amp using an active tone control, and it didn't go down at all well with most experienced guitar players.

The two tone stacks shown above are typical only. There are many variations between different models, but all have fairly similar overall characteristics. There is no true flat setting, and the midrange control only reduces the depth of the notch, the frequency of which varies with control settings. The controls are interactive, so changing the treble will change the notch frequency and affects the bass to some degree. The bass control has less interaction with treble and mid, and the mid control is fairly subtle.
Insertion loss with all controls centred is much greater with the Fender style (average about 15dB) than the Marshall (about 8dB), and the Fender circuit has more boost for both bass and treble. The notch (which varies from around 300Hz to 1kHz for both) is deeper in the Fender circuit. There is no doubt that the two circuits will sound quite different, but that doesn't mean that a good guitar sound can't be obtained from the two. Many guitarists have a preference, but that's often because a particular amp brand is preferred. There are many other guitar amps, and they nearly all use variations of the two circuits shown. It would be silly for me to even try to show all the different circuits because there are so many.

The two response graphs shown above are with the controls set at 50%. Because there's often a mixture of linear and log pots, this doesn't relate directly to any knob setting. The midrange scoop is clearly seen in both traces, and this is one of the main features of tone stacks in general. I don't know of any stack that has eliminated the midrange scoop. Only the frequency and depth change.
These controls are easily modified by changing cap values. There is no design process involved, it's purely a case of trial and error, and ultimately it's all about getting the desired sound. What the controls actually do to the response is secondary to what it sounds like. If it does what the player needs then it's good, if not ...
This type of equaliser is almost only ever used by DJs, and it's quite common in DJ mixers. You will rarely see it elsewhere, but if you were to build a 4-way active system based on Project 125 (a 4-way active crossover) you get this ability free. A frequency isolator is usually simply a 3-way crossover network with its outputs summed to return to a flat response. Project 153 describes a 3-band, 12dB/octave, variable frequency isolator, and if you want to see the full version please refer to the project article. The version shown below has fixed frequencies, and although this may seem quite limiting it's often as much as you are likely to need. The term itself is something of a misnomer, in that you can't really isolate the frequency bands because they have a finite rolloff. You can use 24dB/octave filters (as found in Project 125), but that's generally not necessary to get the effects needed.
To be able to get a flat response without having to bypass the equaliser, the filters must use a Linkwitz-Riley alignment. If Butterworth filters were to be used, there would be +3dB peaks at each crossover frequency (410Hz and 4.1kHz) when the pots are all set the same.

The circuit is simply a 3-way crossover, with the outputs summed. When all pots are set to the same level, the summed output is flat, and the pots let the user turn the level of any band up or down. As shown the frequencies are 410Hz and 4.1kHz, but they are easily changed by changing cap values. The multiple feedback filter (U2) used in the midrange circuit reduces the opamp count. Because it's an inverting stage it means that a separate inverter isn't needed for the midrange. It also is a nuisance because the caps are not the same as those used in the high pass filter (U1) and changing frequencies is more difficult. The alternative is to use a Sallen-Key filter like all the others, followed by an inverter.
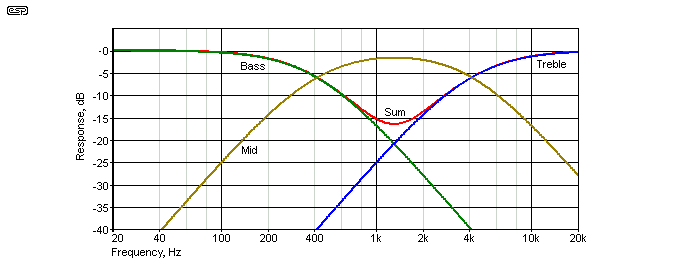
With the circuit shown, the gain is 0dB with the pots all set for 50%, and the summed response is flat to better than 0.1dB. The summed response is shown above with bass and treble at 50% and midrange at zero. This is just an example using fixed frequencies, but of course there are many other possibilities. This type of equaliser is not intended to correct the frequency response, they are used by DJs as an effect.
Finally, there's one last tone control arrangement that was popular for perhaps 5 minutes or so, sometime in the 1970s. It was used in at least one Quad preamp as well as a couple of others, but it died out fairly quickly because it's not really very useful. The effect was to literally tilt the frequency response, so if the bass is boosted, the treble is simultaneously reduced and vice versa. I'm not entirely sure why anyone thought this was a good idea, but it's part of tone control history, so it's included. There are many possible tweaks that can shift the centre frequency or provide asymmetrical response, but these are generally as useless as the circuit itself.
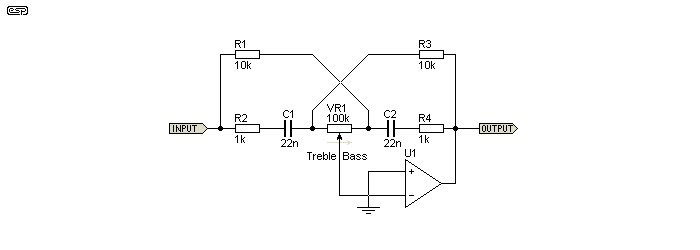
The circuit is straightforward, and uses a frequency selective network wired in reverse phase for high and low frequencies. When one end of the spectrum is boosted, the other end is cut. When the pot is centred, the response is flat. The following response graph shows the response at 25% intervals of the pot. Despite not being very useful overall, there are quite a few different versions on the Net. All behave more-or-less equally, but the Quad version was limited to ±3dB unlike most you will see (including the one shown). To reduce the range, resistors are used in series with each end of the pot (VR1).
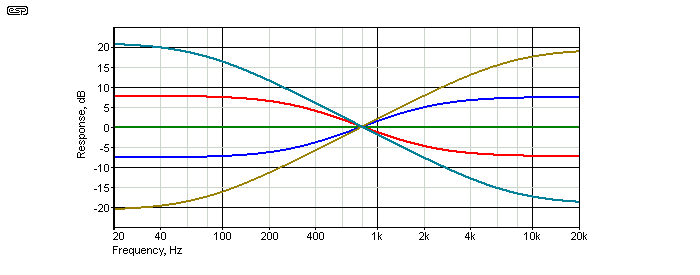
The circuit would be more useful (or maybe less useless) if the range was restricted to perhaps 6dB of maximum boost or cut, but the same thing can be done with more conventional tone controls, and that allows bass and treble to be boosted (or cut) by different amounts to balance the overall sound. As noted, only a few manufacturers decided to use this type of EQ, and it was short lived - presumably because the buying public didn't like it. I expect it seemed like a good idea at the time, but it's really a rather pointless waste of parts. As you may have gathered, I don't recommend it.
I have seen it suggested for a reverb tank, but it's still not as versatile as a set of 'proper' tone controls. For a system with the minimum of knobs it might be alright, but IMO it's still a waste of parts.
In the early days of electronics, it wasn't possible to make a 'gyrator' with any pretense of having a decent Q factor. While it is possible, the gyrator hadn't been invented during the valve era. Back then, inductors were much more readily available than they are today, and would have been cheaper than a valve circuit which probably wouldn't have worked as well anyway. Even into the late 1960s and early 1970s, graphic equalisers often used inductors and capacitors to provide the filter networks. While they worked (and often very well indeed), the inductors were very sensitive to external magnetic fields. If the equaliser wasn't located well away from anything with a power transformer, hum was inevitable.
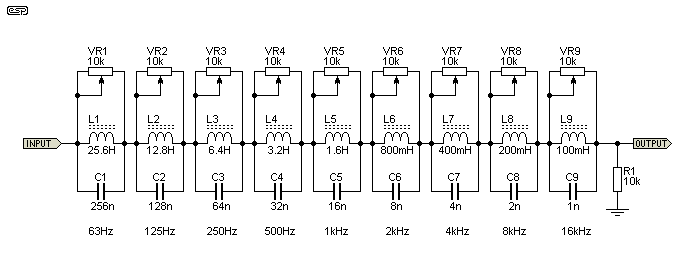
The drawing shown above is a simplified version of one made by White Instruments (Model 4220). This type of equaliser is intended for cut only - allowing 'offending' peaks to be removed. Note the inductor values - the largest (63Hz) is 25.6H - that's a lot of inductance, and it will need a fairly large core to prevent saturation. The load resistor (R1) is critical, and with the design shown it's 10k, which includes the input impedance of the following equipment. If that had an input impedance of 20k, then R1 would have to be changed to 20k (the two in parallel give 10k).
With the values shown, the Q of each stage is about 0.74, more-or-less as required for an octave band equaliser. With any pot set for maximum resistance, the response dip is 6dB, although this can be increased by reducing the value of R1. However, this changes the Q of the filters! Likewise, increasing R1 means less maximum cut at any frequency. The circuit must be driven from a low impedance source, ideally less than 1kΩ. The original design allowed 10dB cut for each filter, which can be achieved by increasing the pot values (VR1 to VR9) to 22k. However, that will increase the ripple in the frequency response when two or more adjacent filters are both set for maximum cut.
The complete design process for the filter networks is outside the scope of this article. As they are simple L/C filters, the inductive and capacitive reactance at resonance will be equal, in this case both equal to the pot value (10k). The formulae shown earlier (under 'Graphic Equalisers') works, but the final Q is affected by the pot in parallel with the tuned circuit. If you use the formulae shown, 'X' is equal to one (1).
In some cases, passive 'notch' filters may be used, especially for guitar, where a reduction of midrange is provided. While the same can be done with hi-fi, it no longer qualifies as 'hi-fi' because so much is missing. A common approach is a bridged-tee filter, which is somewhat less radical than the twin-tee filter used for distortion measurements.

The drawing shows the general configuration of a bridged-tee filter. R1 and R2 don't need to be the same value, but as shown the notch frequency and depth depend on the setting of VR1. At maximum resistance, there's around 1.7dB reduction of the midrange, centred on ~300Hz. As the resistance of VR1 is reduced, the notch gets deeper and the frequency increases. At 50% (25k), the frequency is 400Hz, and the notch depth is 4dB. Things get serious at minimum resistance, with a frequency of about 1kHz and a depth of 28dB.
All parameters can be changed by adjusting resistor and capacitor values. It would not be sensible to attempt to show all possibilities because there are so many. With a fixed resistance for VR1 (say ~3kΩ), R1 changes the notch depth with little effect on the centre frequency, and R2 alters the frequency with little effect on the notch depth. If this arrangement appeals to you, you'll have to experiment with the values - you can use pots in place of R1 and R2 to experiment. C1 and C2 can also be changed, with C1 affecting high frequency performance, and C2 affecting low frequencies. Changing either also affects the notch frequency. It's safe to say that everything affects everything else. No component can be changed that doesn't affect the overall response, but some are subtle, others not.
The bridged-tee circuit must be driven from a low impedance, and the following stage must be high impedance. An input impedance of 1MΩ is recommended for the following stage. This isn't a circuit that you'll see in an equaliser very often, but some guitar/ bass amplifiers include a 'contour' control which is often a variation on the basic scheme shown.
It's fair to say that with the ready availability of opamps, tone controls with greater flexibility and more usable features became possible than were ever available before. When a modern design is set for flat response, there is virtually no change to the signal at all, other than a truly tiny amount of added noise and distortion which is inevitable with any active circuit. Earlier designs could also be fairly flexible, but at the expense of many components (including inductors), and frequencies that could only be switched rather than continuously variable.
When it comes to wide range, flexible EQ, opamp circuits simply cannot be beaten by any earlier technology, despite any contrary claims you might hear. Using DSP is the next level, but there are still many people who prefer to keep signals in the analogue domain if possible. Controlling a complex filter using a touch-screen may be 'high tech', but it's often very hard to beat the feel of knobs on high quality pots. Rotary encoders can be used with digital systems, but you usually lose the ability to see the settings by looking at the knob pointers.
Analogue circuits have another major benefit - they can be built by anyone who can use Veroboard and a soldering iron, or mount parts on a PCB. This isn't even an option for most digital systems unless the person building the circuit can not only solder surface mount parts, but also knows how to program a DSP. There's another disadvantage to the digital approach, and that's IC continuity. Many modern digital ICs (DSPs, ADCs, DACs, etc.) have a short production life, so if the IC fails after a few years it may be impossible to replace. In contrast, opamps have been with us for many years, and there's no indication that any of the popular devices will disappear. Even if an opamp does become unavailable, you can be sure that a suitable replacement with equal or better performance can be found easily.
Whether you like the idea of EQ or not, it's inevitable that it has been used during the production of the original recording. There may be a very small number of tracks that have been created as direct to tape or hard disk without any processing, but they are few and far between. If such material is not a genre you even like, then there's no point at all. In general, EQ will hopefully be applied only where necessary, and preferably with as little change as possible. However, many producers will abuse your senses and the recording by manipulating frequency response so that even more compression can be added without turning the music to mush. Regretfully, this seems to be a popular pastime  .
.
Phase response wasn't even mentioned in any of the descriptions, because it's extremely variable. All equalisers cause phase shift, and the change of phase is much more rapid with a high Q circuit. We can hear the frequency response variation caused by any equaliser, but the phase shift is not audible. There is any number of people who claim that phase is audible, but the claim belies the fact that most programme material has had at least some equalisation, and therefore has phase that varies from the original either for particular instruments and/or for the complete mix. No double-blind test has ever shown that phase shift is audible, provided it's static. Varying phase shift is used to create vibrato (cyclically varying pitch) which is audible, and is used as an 'effect' with many electric musical instruments.
 Main Index Main Index
 Articles Index Articles Index |