|
|
| Elliott Sound Products | Power Factor |
Main Index
Lamps & Energy Index
Based on the number of emails I receive and the astonishing number of websites that provide erroneous or inaccurate information, power factor must be one of the least well-understood concepts in the electrical field. I have seen weird analogies with horses pulling rail carts, glasses of beer (no, I'm not kidding) and countless vector diagrams, all trying to explain the concept [ 1 ].
Most fail, and I have no doubt that I will probably be no more successful than anyone else trying to explain a concept that is impossible to visualise, and only makes complete sense when you understand it. There is a circular reference there - you won't make sense of it until you understand it, which can't be done until you can make sense of the concept. I think you see the conundrum.
However, I shall persevere. I can promise no vector diagrams or horse-drawn carts, but you might want to consider the beer - not as an analogy but as a calmative 
. However, there are two diagrams that I hope will help - at least a little.
In essence, if you have a poor power factor (either from a single appliance or the whole building), you will draw more current from the mains than you actually use. Enough loads with a poor PF place an additional burden on the energy supplier's equipment, and larger substation transformers and distribution wiring may be needed. Rest assured - they will not pay for that themselves out of the goodness of their hearts ¹, but will recoup their outlay by charging more.
Therefore, it's up to the users to ensure that all current drawn from the mains is put to work. With simple loads like motors, PFC (power factor correction) is achieved relatively simply, provided the motor loading is consistent. Things get more difficult where the motor load varies, but this is beyond the scope of a simple explanation.
A direct quote from a document I found shows how little regard is paid to nonlinear loads in particular, even though some of the examples given are responsible for very nonlinear current waveforms ... "Loads on an electrical distribution system can be categorized as resistive, inductive and capacitive. Under normal operating conditions certain electrical loads (e.g. transformers, induction motors, welding equipment, arc furnaces and fluorescent lighting) draw not only active power (kW) from the supply, but also inductive reactive power (kVAr)." (Source: NHP - Power Factor Correction). There isn't a mention of any nonlinear effect anywhere in the document, other than a passing reference to harmonic distortion! However, there appear to have been some changes in the document, and there a bit more information about nonlinear loads (but they are not described specifically as such).
In its simplest terms, power factor (PF) is a measure of how effectively electrical power is being utilised by a system. It can vary from zero to one, and the higher the number the better. Only one component can produce a PF of zero - a capacitor. It absorbs current with each half cycle of the mains, then gives all of it back with no work being done. A high quality capacitor is as close to an ideal reactive component as we can get. Interesting, but not terribly useful.
You can (if it helps) consider that power factor states "the degree to which a load matches a pure resistance".
It's interesting and somewhat depressing that this article was written in 2012, and as of 2020 virtually nothing has changed on the Net. Descriptions of power factor are still (mostly) referring to the simple cosφ (the cosine of the phase angle difference between voltage and current) formula, and in many cases there is still little or no mention of nonlinear loads. There are videos and countless pages describing power factor, and many of them are either useless (because they don't mention nonlinear loads) or in some cases just plain wrong. Given the fact that we now have more nonlinear loads than ever before, it's a sad state of affairs that there is so little factual information about the effects of a distorted current waveform.
It's also very misleading to categorise transformers as presenting an inductive load. While an unloaded transformer (as used by hobbyists and in small systems) with nothing connected to the secondary is largely inductive, the magnetising current is nearly always nonlinear, and the inductance of a transformer is usually very high. Its inductive contribution is generally quite small due to the low current, and rarely needs more than a few microfarads (at most) to get the voltage and current in phase. Consider a fairly typical 250VA transformer, which will have an inductance of perhaps 30 Henrys (assume linear magnetising current - an 'ideal' transformer). It will draw a magnetising current of 24.5mA at 230V, 50Hz, and current will be perfectly in phase with the voltage with a parallel capacitance of only 340nF. Once a load is applied, the power factor is determined primarily by the load, and not the transformer.
With a 230W resistive load, the transformer's phase angle is around 10.4°, a power factor of better than 0.98 with no PFC capacitor. This is already an excellent figure! With 240nF in parallel with the primary, the power factor is unity at any load. Interestingly, placing the capacitor in parallel with the secondary works just as well, provided it's adjusted to match the turns ratio. For the example, if the transformer is 10:1 (23V output with 230V input), a capacitor on the secondary has to be 34µF (the value is adjusted by the square of the transformation ratio). Real transformers behave a little differently, because their magnetising current is usually not a sinewave.
The true power factor of a transformer is the reflected load on the secondary. If this is a rectifier and capacitor bank (the most load for many applications), the secondary current is nonlinear, and therefore, so is the primary current. Thus, a transformer is not an inductive load, it nonlinear. For reasons that remain deeply mysterious, this barely rates a mention - anywhere!
While this also applies to power distribution transformers, these are usually the responsibility of the power company, and there's not much that can be done at the 'user side' that will correct the power factor if it's reactive (which means either inductive or capacitive). While it is certainly possible for a user to correct the reactive PF of the incoming mains, doing so is not sensible and would provide no benefit. Users need to correct the PF of their equipment (where possible), and no more.
First and foremost, we need to define power. Power is the rate of doing physical work performed by (or absorbed by) an electrical machine (the load), determined by the voltage across the load and the (in-phase) current through that load. Actual power with DC always follows this simple definition. Work can be a mechanical function (commonly rotary, such as with motors), or the production of heat. It doesn't matter if heat is the desired work or a by-product of inefficiencies in the electro-mechanical system. Efficiency is determined by input power vs. output power. If the desired output is rotary motion, heat generated by the machine only contributes to the power input, and is a 'waste product' (it contributes no rotary motion).
Work and energy are measured in Joules while the rate of doing work is measured in Watts (or multiples thereof). A Watt is one Joule per second. Watts represent real energy, and that's how you are charged by your electricity supplier. Many industrial customers are penalised if the power factor at the connection point is less than a predetermined minimum (typically ~0.9, but it varies). The new smart meters that are being installed worldwide are also (apparently) capable of charging residential customers for a poor power factor if legislation ever allows it.
Unless the reader is familiar with electrical and electronic concepts, defining power is actually harder than it sounds. As noted, power is usually defined as the product of current and voltage, and for DC it works every time. If we have 10V across a 10 Ohm resistor, it will draw 1 Ampere and dissipate 10 Watts. There is no ambiguity - the answer is as accurate as the voltage and current readings will allow.
Where things get complex is when we no longer have a DC power source. Alternating current (AC) is supplied to business and domestic users worldwide, although it's outside the scope of this article to explain the very good reasons for this. Suffice to say that this is the case, with power delivered at a frequency of either 50Hz or 60Hz at a variety of voltages. Both frequency and voltage are consistent in most countries, and typical combinations are 230V RMS at 50Hz (most of the world, including Europe and Australia), or 120V at 60Hz (the US and Canada, and a few other regions).
Provided the load is still a resistor (as above), 10V RMS across a 10 ohm resistor will still cause 1A to flow, and the resistor will dissipate 10W. The frequency and waveform are (surprisingly) irrelevant. Root-Mean-Squared (RMS) voltage measurements mean that the RMS voltage will cause identical heating in a purely resistive load as an equal DC voltage. 10V RMS is exactly equivalent to 10V DC - but only with a completely resistive load (e.g. incandescent lamp, heating element, electric kettle, toaster, etc.).
The simple loads referred to above (plus many others of course) are never a problem. Power is calculated as the product of voltage and current ...
Power = Volts × Amps
There is no ambiguity, and the answer is always right.
Naturally, there are countless machines and appliances that are not a simple resistor. Motors (large and small) and discharge lighting (fluorescent, metal halide, mercury vapour, etc.) have been the traditional 'problem' loads in the past, but there are now many loads - some extremely large - that are nonlinear. The applied voltage is a (nominal) sinewave from the mains, but the current drawn by the load is not. This causes problems that cannot be calculated by the 'traditional' formula and cannot be corrected using otherwise tried and true methods.
Herein lies the problem!
I don't know why, but it seems that perhaps 75% or so of electrical engineers are still stuck firmly in the past, and fail to understand (or comment on) power factor as it applies to nonlinear loads. Most rely on an old short-cut formula that considers only one thing - phase angle. The correct, and ideally the only way to determine power factor is to use the right formula ...
Power Factor (PF) = Real Power / Apparent Power
Real power is that which is measured by a wattmeter, such as the one in your meter box. Real power is always measured in watts, and was previously considered to be that part of the supplied mains that performs work. The reactive part of the current waveform is 'returned' to the grid when a motor (for example) is running, and you are only billed for that fraction of the current that is used to perform work. 'Apparent' power is the product of RMS voltage and RMS current - volts × amps (VA). Unlike the DC condition, VA is no longer the same as watts with AC mains!
Note: To measure 'true' power with AC, you need a wattmeter. There is a project for a wattmeter to measure true audio power (see Project 189), and that can be adapted for use with mains. However, this is not recommended, because everything is at mains potential. A better solution is to use a wattmeter module, as described in Project 172. The audio version is useful reference material, and shows how it's done (albeit using analogue techniques rather than digital).
Modern switchmode power supplies have changed the definition, because they are not reactive, and nothing significant is (or can be) returned to the grid. There is no out-of-phase component in the current waveform, but the current waveform is often highly distorted and rich in harmonics. Countless websites and/or engineers will try to claim that these supplies are somehow reactive, and any explanation that claims any significant (measurable) reactance is quite simply wrong. Not just woefully inaccurate, wrong!
| In reality, there actually is a tiny amount of reactance caused by the EMI (electromagnetic interference) filter. However, its influence is very
small indeed, the mains waveform is not materially affected, and any 'phase angle' that may be introduced has absolutely no bearing on the overall power factor. The power factor is
changed by such a tiny amount by the addition of the filter components that it's not worth considering. The difference can be measured on a simulator, but is unlikely to even register
on typical test instruments. I mention this purely in the interests of completeness, and to save people the trouble of complaining that I had failed to address it. 
|
A nonlinear load such as that shown in Figure 2 may draw 2.8A from a 230V supply and deliver a power of 296W. The RMS current drawn (based on a similar simulation) will be 2.8A. This gives a VA rating of 644VA, so determining the power factor is based on the following formula ...
Power Factor = W / VA
Power factor = 296 / 644 = 0.459
An interesting formula is shown in the article Power factor from Wikipedia (which is otherwise IMO not particularly well explained or genuinely useful). It refers to 'distortion power factor', another way to describe the effect of a nonlinear load. Unfortunately, measuring distortion of the mains current waveform is much harder than measuring the RMS voltage and current, and it assumes that the input voltage waveform is a pure sinewave, which is rarely the case. Using this formula is reasonably accurate, but is not as good (or as easy) as the accuracy you obtain by measuring 'apparent power' (VA) and 'real power' (watts) and using the formula shown above.
Power Factor = 1 / ( √ 1 + THD² )
In the above, THD is the total harmonic distortion expressed as a decimal value, e.g, 50% THD would be expressed as 0.5 in the formula. If we use an example circuit such as the nonlinear current waveform shown in Figure 2, the distortion will be about 180%. Applying the formula gives ...
PF = 1 / ( √ 1 + 1.8² ) = 0.485
This compares well with the value that's obtained by measuring the RMS voltage and current (VA) and the actual power as measured with a true power meter (watts). As noted though, while it works, it's much harder to measure THD than it is to measure volts, amps and power. This makes it interesting, but not particularly useful for 'real world' applications. It's made less useful by the fact that the 'typical' mains waveform already has a THD (total harmonic distortion) of between 5% and 10%, and it's difficult to factor that into the equation.
| Watts Versus Volt-Amps | ||
| Watts | VA | |
| Power Type | Real | Apparent |
| Calculation (DC) | VDC × IDC | VDC × IDC |
| Calculation (AC) | ∫ V(t) × I(t) Δt | VAC × IAC |
| Used For | Calculating heat generated/ dissipated & calculating energy cost | Sizing cables, fuses & circuit breakers |
| To add together | Add Wattage Linearly | Not easily done ¹ |
| Measurement Instrument | Wattmeter | RMS Multimeter |
Note ¹ - While VA ratings can be added, the result may not represent the true value. In many cases, linear addition will provide a figure that's higher than the actual figure, but it will never be less. This is true whether the loads are reactive or nonlinear. For DC, there can be no reactive power, but nonlinear (e.g. pulse current) requires that the meter used correctly averages the current. This will usually be true, but it's not guaranteed. Some digital multimeters may not perform averages well, especially if the current changes slowly.
There is a simple thought experiment that I hope will give you and idea of both reactive and nonlinear loads. I've not seen this one used before, but I'm hopeful that it will convey the idea better than some of the more traditional explanations. If not, I apologise in advance.
Figure 1 - Reactive Power, Nonlinear Power & Work
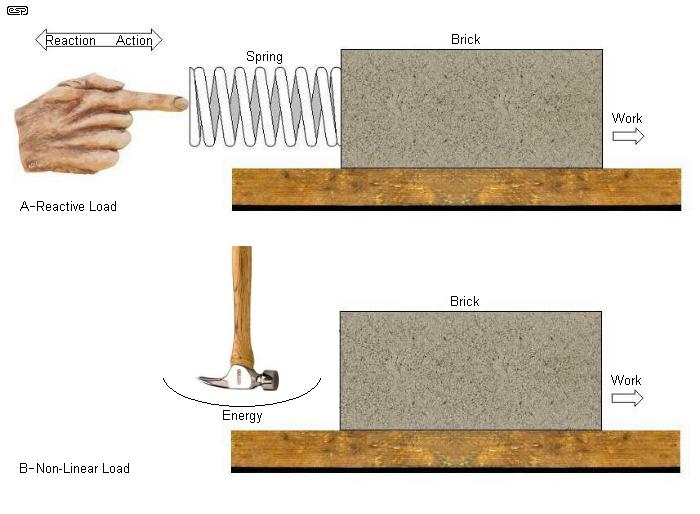
The diagram shows the general principle of reactive power (A) and nonlinear power (B). Imagine that the surface is slippery, and there's no 'stiction' (static friction, where it takes additional force to get something to start moving [ 3 ]) With the reactive case, your task is to push the brick along the plank of wood, but you have to do it with little pulses of energy, all exactly equal and preferably at 100 or 120 times a second. When you push, the spring compresses and the brick moves forward a little (some work is performed). As you release (this is AC, remember), the spring will return some of the energy you used. It's not useful and performed no work, but you had to expend the energy to compress the spring, and absorb the energy it returns to you. This is a reactive load, and the power factor is determined by how much energy is used to perform work, versus how much is just absorbed and returned by the spring.
So, when the load is reactive (with the spring) not all of the energy you expend (incoming power) is converted to work - moving the brick. This system has a poor power factor. If you remove the spring, all of the energy you put in will move the brick, as long as it's enough to overcome friction (resistance). In this case, there is no reaction (energy return), and thus approximates unity power factor. In an electrical machine, you don't have the option of simply removing the spring if you don't like it, because it's part of a circuit that can't function if it's not there.
Should you decide to use a different circuit, you can move the brick with a hammer (again, swing it back and forth 100 or 120 times a second), there will only be a power transfer in the brief period when the hammer strikes the brick at the peak of the swing (please ignore any rebound - that's a physical phenomenon that's not part of this thought experiment). The remainder of the swing is basically 'idle-time' when no useful work is performed. So it is with the mains - nonlinear loads may only draw current for a few milliseconds each half cycle. The rest of the time the mains voltage still swings back and forth much like the hammer, but converts zero energy into work - namely moving the brick.
To see the electrical waveforms, have a look at Figure 2.
Apparent (aka 'reactive') power is only possible with AC, and used to be the result of reactive loads - most commonly motors, but also includes traditional iron-core ballasted discharge lamps. More recently, we have had more and more nonlinear loads, such as computer power supplies, compact fluorescent lamps (CFLs) LED lighting and DC plug-pack power supplies. Even standard transformer based power supplies used for hi-fi amplifiers are nonlinear. There are also much larger nonlinear loads, such as inverter technology air-conditioners and microwave ovens, induction cooktops and many other products (TV sets, home theatre systems, etc.). Indeed, there is an almost infinite number of appliances small and large using switchmode power supplies. A large majority of these switchmode supplies draw a highly nonlinear mains current, commonly so different from the voltage waveform that it's sometimes hard to imagine how the supply network survives.
Many industrial machines also draw nonlinear current, and these machines can be very large - massively so (think of electric arc furnaces for example). Even more traditional high-power loads such as 3-phase rectifier systems (used to power electric trains and other large DC motors, electroplating tanks, etc.) present a very unfriendly distorted current waveform back to the grid. Being distorted, the current waveform is rich with harmonics ready to cause havoc. Again, the standard phase-based (cosφ) power factor calculations cannot be used with these nonlinear loads.
With AC, apparent power is defined as the product of voltage and current (RMS for both). This gives us a figure that's called VA (volt-amps), and it may or may not be the same as the power measured by a wattmeter. VA can never be lower than the power in watts, but with purely resistive loads they will be exactly the same. This is a power factor of unity - the best that can be achieved. This means that the voltage and current are not only in phase, but have the same waveform (nominally a sinewave). There is no 'excess' or 'wasted' current.
Traditionally, the favourite formula has always been that PF = cosφ - the cosine of the phase angle difference between voltage and current (also known as 'displacement' power factor, because the voltage and current waveforms are displaced in time/ phase). It's a nice short-cut that only works with undistorted sinewaves and linear reactive loads. The simple fact is that a vast number of electrical loads are nonlinear, and the formula doesn't apply - it can't apply, and it is nonsense to imagine that a formula that applies only to clean sinewaves is appropriate with any nonlinear load.
Figure 2 - Reactive Power, Nonlinear Power, Voltage & Current Waveforms
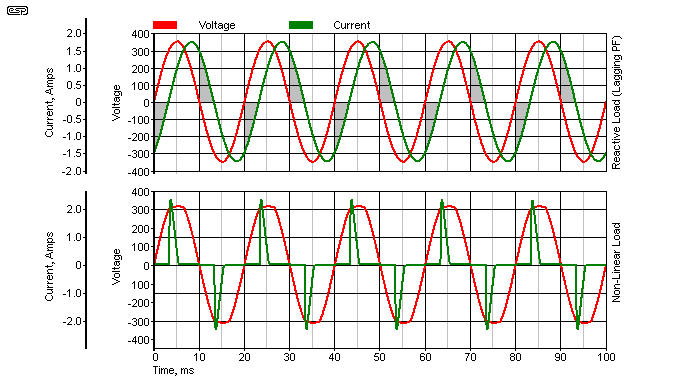
Above, we can see the two main kinds of load - reactive (top) and nonlinear (bottom). The reactive load's current is not in phase with the voltage, and just like the spring in Figure 1 (A) fails to keep the pressure (voltage) and displacement (current) working at the same moment in time. The same happens with a reactive load. The current lags the voltage by a number of degrees, and we can work out the power factor by taking the cosine of the phase difference. As noted though, this only works with reactive loads that have no nonlinear function. We can also have capacitive reactive loads, but they are much less common and won't be covered here.
|
| In particular, look at the upper waveforms for the reactive load. There is a point during a cycle where the voltage is positive while the current is negative (and vice versa). This is shown by shading at the relevant parts of the waveform. For any load to be considered reactive, this condition must exist during each cycle. If it doesn't, then the load is not reactive. This is an important concept to understand. Knowing this instantly allows us to look at the lower nonlinear current waveform and note that these prerequisite conditions for a reactive load do not exist! When the voltage is positive, so is the current, (and vice versa), and there is no point in the waveform where they assume opposite polarities. |
The lower (nonlinear) waveform is created by a circuit such as that shown next. You might imagine that if there's a transformer between the mains and the rectifier, filter and load that things would be different. They're not - the transformer (as used in almost every 'linear' power supply) simply reflects the load waveform back to the primary, and the mains 'sees' the same waveform. It is modified (very slightly) due to the transformer's inductance, but the general principle is unchanged.
Figure 3 - Nonlinear Power Supply Example
With a typical nonlinear load, the voltage causes no current flow at all until it almost reaches the maximum. There's a short burst of current and then nothing until the next half cycle. The traditional formula does not work with a load such as this. Not even a little bit! A vast number of loads combine both reactive and nonlinear functions. A perfect example is a traditional fluorescent tube and magnetic ballast, which can never be corrected for a unity power factor. One can get close, but the nonlinear current prevents total correction. The typical best case power factor for a fluorescent lamp and ballast is around 0.9 - all other discharge lighting is similarly affected.
Until such time as the 'old-school' electrical engineers of the world wake up to reality and understand that their favourite formula does not work with nonlinear loads, we will continue to see non-sensible replies to forum posts, completely inaccurate claims being made and expensive reports that totally fail to address the real issues. There are many otherwise excellent papers about power factor and the benefits of power factor correction (PFC), but some fail to even mention the 'elephant in the room' - nonlinear loads. Some of these reports (they are in the minority) will mention harmonic currents - these are the direct result of nonlinear loads, and cannot be created by any truly linear load.
By definition, a reactive load both absorbs power from the source (e.g. the supply grid), and (most importantly) returns at least a portion of the power absorbed back to the source. A nonlinear power supply does not satisfy this criterion, regardless of whether there is a capacitor following a diode rectifier or not. It seems that many people imagine that the load (which includes the filter capacitor) is capacitive, but this is simply not true. The diodes preclude any possibility of power being returned to the source, and the capacitor is irrelevant.
Some (but not all) commercial/ industrial power factor correction systems include harmonic filters that are designed to filter out the harmonics generated by nonlinear loads. A system that's designed to correct an overall factory or commercial building where the power factor is lagging (inductive loads) cannot correct for nonlinear loads unless it includes harmonic filters.
In a nutshell, poor power factor causes a load to draw more current than it needs to convert into work. It doesn't matter why the power factor is bad - the result is that the grid has to supply more current than the equipment actually uses to perform the work. Some poor power factor loads can be corrected - either near the machine responsible or elsewhere, and others cannot be effectively corrected at all. Motors and iron-core ballasted lighting equipment are easily corrected, although with discharge lighting systems there are actually two reasons for the bad power factor. As mentioned above, all (magnetically ballasted) discharge lighting causes a lagging (inductive) power factor, but the current is also nonlinear. This means that it is impossible to get unity power factor, because only the inductive component can be corrected.
Nonlinear loads cannot be corrected with any conventional PFC system. The current drawn from the mains is distorted, and there is very little that can be done externally to remove the distortion. While it can be done, it is a difficult and expensive exercise, and very few external correction systems currently exist. The best way to solve the problem is to use more sophisticated power supplies that incorporate active PFC (see active power factor correction for a much more complete analysis of power factor correction techniques).
If a load has a power factor of 0.5, that means it will draw twice as much current from the mains compared to an equivalent load with unity power factor. A 230 watt appliance with a PF of 0.5 will draw 2A from the mains (460VA), while only performing useful work amounting to 230 watts. In most cases, householders are not billed for the extra current, only for the power used - 230W in this case. Most large industrial users are charged for poor power factor, and there is a financial incentive to make it as good as it can be.
Since so many loads today are nonlinear, the old cosφ formula is useless - it can only ever create wrong answers. I've seen laboratory reports claiming that a LED lamp has a power factor of (for example) 0.85 ... leading! What rot! The measured power factor is due to nonlinearity, not phase shift. It's neither significantly leading nor lagging - it's nonlinear, and the sooner certified test labs and official standards are brought into the 21st century the better. The traditional formula doesn't even work properly with many 'legacy' lighting systems. As already noted, all discharge lighting creates a nonlinear current waveform that cannot be corrected - even if the voltage and current are perfectly in phase!
So, having a power factor of less than unity simply means that the appliance (whatever it might be) draws more current from the mains than it can put to effective use. It really doesn't matter why (when only the PF is being quoted), but by knowing about it an electrician can take the extra current into account when running cables for an installation. It's no longer possible to just look at the power rating - just because an electronically ballasted lamp (for example) is rated for 23W doesn't mean it only draws 100mA from the 230V mains.
If the power factor is 0.5, each lamp will draw 200mA, so an 8A lighting circuit can only handle 40 of them - not 80 as might be imagined. The same applies for any electrical device with a PF of less than 1. Note that for a variety of reasons, it may only be possible to use perhaps 10 of the hypothetical lamps mentioned above on an 8A lighting circuit, especially if all lamps are to be turned on at the same time (with a single switch for example). This is an issue that has already caused grief in some modern lighting installations. CFLs (compact fluorescent lamps) have really lowered the standards, with many struggling to manage a PF of 0.5 - and some are worse. These are nonlinear loads that have a current waveform that looks remarkably similar to the one shown in Figure 2.
One often-repeated claim is that power factor is a measure of a machine's 'efficiency'. This is misleading in the extreme, and is a completely false representation of the word. Efficiency is a measurement of power in (energy consumption) vs. power out (work), and power factor generally has zero influence over that. Correcting the power factor of a machine (e.g. an electric motor) does not improve its efficiency one iota, nor does it make the motor run cooler (another completely false claim that you may see).
Note: Power factor correction does improve the overall efficiency of the power network, because the relationship between kVA and kW is closer to unity, so the full capacity of the distribution network can be utilised.
This short article is intended as a primer into the mysterious world of power factor. It is quite deliberately devoid of vector diagrams, complex formulae or other distractions, and will hopefully give the reader at least a basic understanding of the topic. The most important message is to forget the cosφ formula, because as long as people keep using it they will most often get a nonsense answer.
There are innumerable sites on the Net that will also describe power factor, but the vast majority completely omit any reference to nonlinear current waveforms and naively assume the only sources of a poor PF are motors and other inductive loads. Energy suppliers (who should know better) are just as guilty of this as anyone else. Whole reports [ 2 ] have been written (no doubt at great expense) that have totally ignored nonlinear loads. Not a mention. Nothing!
The article Active Power Factor Correction has a great deal more information, and there you will find detailed graphs, waveforms and a detailed explanation of PFC in general and active PFC systems in particular.
In case anyone is wondering why I have used so many examples of lighting products, that's because lighting is generally considered to be the largest single user of energy [ 4 ] of all. Inefficient lighting also has other flow-on effects, because additional cooling is often needed to remove excess heat generated by lighting systems, adding to the overall impact.
Overall, there is a net gain when (for example) incandescent lamps are replaced by LED (or even CFL) lamps, because although the power factor may be much worse, their current draw is far less and the power consumed is also lower. Many of the latest LED lighting products (mainly high power fittings, but some lower power tubes/ fittings as well) are now more likely to use active PFC, so not only do they draw far less current, but also present a friendly current waveform back to the supply grid.
There are a great many companies who sell (expensive) automatic PFC equipment, but only a few will mention nonlinear loads. There is definitely a benefit to installing a PFC cabinet if your business operates many motor-driven machines, but if those machines are fitted with VFD (variable frequency drive) then the mains loading is likely to be nonlinear unless the VFD utilises active PFC in its circuitry. Adding a 'traditional' switched capacitor PFC system will achieve nothing useful in either case.
Transformers are regularly cited as a contributor to poor power factor, with their magnetising current taking most of the blame. This only applies when the transformer is lightly loaded. At any reasonable load, power factor is usually excellent, provided the load on the secondary of the transformer is linear! If the secondary is used to obtain DC (as with amplifiers and countless pieces of industrial equipment), the load is not linear, and low power factor is again the result of the nonlinear load. Traditional PFC systems won't work, and nor will the outdated and pointless cosφ formula.
It is extremely important that novice readers in particular read the article The Great 'Power Saver' Fraud, lest they think that there might be some (financial) benefit if they improve the power factor of their home's electrical products. The devices offered are almost all total frauds, and will not save you a cent. Indeed, if enough people installed these units (which contain only a small capacitor in almost all cases), the grid could be faced with a leading power factor that can't be corrected by most existing substation equipment.
Unfortunately (but not surprisingly), most of the direct links that I included have vanished. A web search will provide similar information, but you'll have to sort the fact from the fiction yourself. Providing references seems to get harder every year, because 'webmasters' never seem to think that anyone might want to see material again. It gets moved (often to very obscure places on the site, or is removed altogether.
Main Index
Lamps & Energy Index