

|
| Elliott Sound Products | Balanced I/O (Part 3) |
 Main Index
Main Index
 Articles Index
Articles Index
This is the third article on the topic of balanced interfaces, and it covers things that don't appear elsewhere. Balanced inputs and outputs are considered essential for many applications, but the common circuits can seriously degrade the CMRR (common mode rejection ratio) without you realising it. The degradation is almost always at the top end of the frequency range, and the primary cause is phase shift within the driving circuits. This article looks at the reasons for degradation, and what can be done to prevent the CMRR from falling at high frequencies.
In reality, some degradation is almost impossible to prevent without the use of precision (and generally high speed) circuitry, where every part of the circuit is optimised carefully. This is needed to ensure that both inputs or outputs have exactly the same propagation delay at all frequencies. If this sounds like it may be hard to achieve, you'd be absolutely correct. For the purposes of this article, the 'audio range' is defined as being from DC to 100kHz. Above 100kHz things get a great deal harder, especially if the response is expected to extend down to low frequencies (DC to a few hundred Hertz).
Despite their generally excellent performance, opamps and other circuitry (such as FDAs) are the primary cause of the issues seen. The CMRR of all opamps is a frequency dependent parameter, and some datasheets specify it at 60Hz, sometimes with a graph showing CMRR vs. frequency. In other cases CMRR is provided as a minimum and 'typical' specification with a defined source impedance, but with no frequency information. This almost always means that it refers to DC or low frequency performance only.
It's important to understand that while the problems described here are very real, most of the time they won't create any issues. This is because the majority of the issues faced are caused by hum loops (aka earth/ ground loops), so the predominant frequencies to be 'eliminated' are mains (50/60Hz) and their harmonics. Even allowing for up to the 17th harmonic, this remains under 1kHz for 50 and 60Hz mains (the 17th harmonic of 60Hz is 1.02kHz).
The issues described affect nearly all balanced input and output circuits, including those described on the ESP website. This shows clearly that the issues described here are not normally a problem at all, but in the interests of providing the most complete information, the problems and their solutions are described in detail. A separate article looks at balanced input circuits based on instrumentation amplifiers (INAs), so only limited info on those is provided here.
For the purposes of simulation and demonstration, TL072 opamps are used throughout this article. This is because they are very common, low cost, high performance devices (although they really don't qualify as 'hi-fi' compared to many far superior devices available today). The main reason they were used is simply for consistency. To be able to show that one circuit is 'better' or 'worse' than another, the number of variables has to be kept to a minimum. It also helps that the simulator I use has a fairly good model for the TL07x series, but does not include many other well known (and especially audiophile) types.
The common mode rejection ratio (CMRR) for the TL07x series of opamps is quoted as a minimum of 75dB with a 'typical' figure of 100dB. By way of comparison, the LM4562 (a 'premium' opamp) has a minimum CMRR of 110dB, with a typical value of 110dB, and the AD797B (very expensive) has a minimum CMRR of 120dB and a typical value of 130dB.
Also, except where noted otherwise, resistor values are considered to be exact. This is unrealistic in the real world, but it helps to highlight issues that are related to the opamp or circuit topology, while ignoring those that are due to component tolerance. At the very least, 1% resistors are essential, but higher precision is necessary if a particularly high CMRR is required. Component tolerance is just as important for balanced drivers (transmitters) or receivers.
It is not the intent of this article to produce circuits (or circuit ideas) that have unlimited common mode rejection, because it's not possible with real-world parts. The idea is to alert the reader to the limitations of common circuits, so that the effects can be mitigated where a design really does need the maximum possible rejection. All circuits are imperfect, but with careful design the imperfections can be minimised. At some point, one has to decide whether the added PCB real estate and/or cost is worth it for the gains realised.
The problems investigated here are based on the real world limitations of opamps. In a simulator we have access to 'ideal' devices, and if these are used everything works perfectly at all frequencies from DC to daylight. Since we can't actually buy an ideal opamp (sad but true), we have to deal with the limitations as best we can. It's usually not too difficult, simply because the frequency range occupied by audio is (not entirely accidentally) the very same range for which most opamps are designed. Note that use of high priced discrete opamps will rarely (if ever) improve anything, and in many cases they will be worse, not better.
You will see here that CMRR diagrams appear 'upside down', and show the CMRR as a negative dB figure. This is due to the way I ran the simulations, but the end result still shows what happens at the frequencies between 10Hz and 100kHz (the range I used for the simulations).
Microphones are mentioned first because they are a 'special' case. It's traditional that mic preamps are balanced, and many people think that it's essential. However, it's not (and never has been) a requirement for microphones, because they are a fully floating source. This significant, because it means that a mic can be connected to a preamplifier using only a shielded lead (coaxial), and there is no noise penalty. There are caveats - a cheap lead without a full braided shield will pick up noise, not because it's unbalanced, but because the gaps in the shield mean that the inner and outer conductors cannot 'talk' to each other properly, and high frequency (e.g. radio frequency) noise may penetrate the gaps in the shield.
Contrary to popular belief, an unbalanced mic cable can be just as quiet as a balanced cable, for the simple reason that the mic has no earth/ ground reference. There are situations where an earth reference may exist - typically involving a human touching both a guitar and a microphone. However, this connection is a relatively high impedance and almost never causes a problem. Despite this, it's traditional that mic preamps feature balanced inputs, but the reason is not to get 'higher performance'.
Back in the 1970s, most PA systems used unbalanced mic inputs. While many had some significant problems, microphone lead induced noise was not one of them. Microphones were overwhelmingly dynamic types, with very few 'exotic' mics in use. High impedance microphones fell from favour quite early - they were common during the 1960s because most gear was valve-based, and gain was expensive. These mics were always noisy, because high impedance circuits are susceptible to electromagnetic interference, triboelectric noise from the cable itself, and circuit noise in the preamp.
A mic is not only floating, but well shielded from external noise, other than hum induced from nearby transformers directly into the voicecoil. A few mics include a 'hum bucking' coil to cancel out noise from magnetic fields. The mic body was then (and still is) earthed via the cable shield, which minimises electrostatic noise pickup. This is the noise you hear if you touch the pin at the end of a guitar or RCA lead. Another noise source was lighting dimmers, but provided no-one did anything silly like bundling lighting and mic cables together, even this usually produced little noise.
Over the years, people started using 'proper' mixers, and even many early versions still had unbalanced inputs. This started to change as performers used more and more equipment, much of which was mains powered. With that came earth/ ground loops, and much hum and noise ensued. Balanced inputs became the standard, to accommodate 'line level' equipment, microphones and phantom power. The latter forced the change, because phantom power is applied between the two conductors and the shield. Maintaining the balanced (and floating) mic capsule means that if a dynamic mic is fed with phantom power (accidentally or otherwise) it won't be damaged because both ends of the voicecoil have the same DC voltage (+48V).
Because mixer inputs were balanced, it became standard for mics to be connected with (now standard) shielded twisted-pair cable, fitted with XLR connectors. Using the balanced connection also allowed the use of phantom powered mics, which is a common requirement with modern stage and studio setups. This change made absolutely no difference to floating signal sources (like dynamic microphones), but meant that all XLR leads were the same and there was no chance of mix-ups when setting up for a show. Despite anything else you may come across describing balanced mic leads, they are not necessary. In fact, if the internal conductors aren't twisted properly, a balanced mic lead can result in more hum pickup (via the cable) than a good quality unbalanced lead. This is exactly the opposite of almost everything you'll read elsewhere.
Balanced line drivers and receivers (as covered below) are needed when mains powered equipment is connected to a mixer (or other mains powered equipment), and the intent is to prevent (or at least minimise) the creation of earth loops. Since this condition cannot exist when mics are used normally, using balanced cables with XLR connectors is a convenience, not a requirement. A low impedance microphone with no connection to ground has little or no common mode noise, as that requires a loop, which is not possible with a floating source.
Consider that many very expensive laboratory microphones use an unbalanced connection. 4mA current-loop (aka ICP, IEP, IEPE or CCP) mics are made by PCB Piezotronics, ROGA Instruments, BSWA, G.R.A.S, Brüel & Kjær, etc. Most are fully calibrated, and rated as Class-I or Class-II, and they are used primarily for sound level monitoring and high-precision measurement work. These are at the very top of the 'tree' when it comes to microphones, and they can use up to 100 metres of unbalanced coaxial cable, terminated with BNC connectors. It should be obvious that if a balanced connection were necessary for low noise, then these mics would be balanced. It's worth noting that the mic and preamp are separate, and there are different mics that can be used depending on usage. Prices are never provided, so you know straight away that they cost more than most of us can afford (think in terms of $many hundreds$). I've worked with these, and they are virtually dead silent, even in the presence of workshop interference.
One of the most common balanced output stages is shown below. This is basically the same as the one used in the PCB version of Project 87B. While it has flaws as described below, its performance is normally more than acceptable for general purpose use, and that was the design intent.
CMRR at DC is almost perfect, reaching better than 100dB, but even by the time the frequency has risen to 1kHz, CMRR is down to 70dB, and reduces at 6dB/ octave as the frequency increases. At 20kHz, CMRR is only 44dB - not complete rubbish, but certainly not wonderful. This is based on the resistor values being perfect, and even 1% tolerance will degrade things further. If there is a 1% difference between R3 and R4, the best CMRR obtainable is 46dB, but at 20kHz it's still 44dB, so things are not quite a dire as they may otherwise appear.
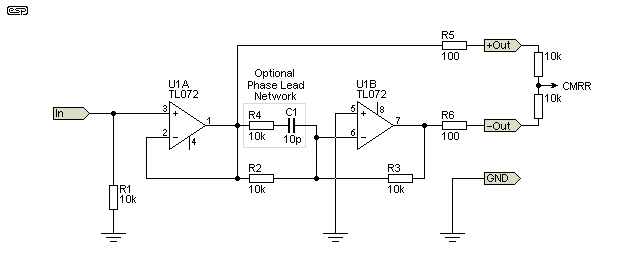
Figure 1 - Basic Balanced Driver Circuit
This is a common circuit, and can be found in hi-fi equipment, commercial (live sound) gear, and almost anywhere that a balanced output is needed. As discussed above, it's not perfect because one signal is delayed by only U1A (which is immaterial in this arrangement), but the other (inverted) signal has the extra delay of U1B. When combined, they are not (and never can be) perfectly matched at all frequencies. The small time delay (aka propagation delay) of U1B plus its high frequency phase shift means that the two signals are not exact but inverted replicas of each other. There will always be small amplitude and phase errors that mean the summed output is non-zero.
The boxed network creates a phase lead for U1B, which improves the CMRR vs. frequency quite dramatically (at least in a simulation - reality may be different), but it may not be easy to get it right and the advantage is (perhaps surprisingly) probably not worth the effort. However, if it is included, the measured CMRR is improved by about 25dB (assuming that all resistor values are exact of course). One thing that is not immediately apparent is the fact that an inverting opamp stage operates with a 'noise gain' of two. While the signal gain is (-) unity, the actual internal gain is x2, so inverting and non-inverting buffers can never be exactly equivalent. The value of R4 is (relatively) unimportant, and is selected to ensure that excessive high frequency boost is not created.

Figure 2 - CMRR Of Output Voltage
The reduction of CMRR with increasing frequency is obvious. It's measured simply by using exactly equal value resistors from each output, shown in Figure 1. If amplitude and phase are equal (but opposite), the result is zero signal at the mid-point of the two resistors. Any deviation of amplitude or phase between the two results in degraded cancellation at the 'CMRR' output.
The 'phase lead' circuit helps to counteract the lagging phase response of U1B, and the resistor and capacitor values depend on the opamp. 10pF is right for the FET input TL072, but opamps with bipolar inputs will require a value to suit (usually larger than 10pF, but unlikely to be more than 47pF). U1A also has a lagging phase response, but correcting that would make matters worse, not better.
You may also imagine that providing the input to U1B directly from the input along with U1A would mean that the two circuits would be closer to being identical, but it doesn't help. The best case CMRR (at DC) is reduced to just under 100dB, and at 20kHz it's only 5dB better than the uncompensated response (green trace above), but is 20dB worse than the compensated version.
If the circuit is re-configured so that both opamps are used with a noise (or internal) gain of 2, the performance can be improved. To give you some idea of how little phase shift can create a problem, consider two perfect sinewave generators, producing sinewaves 180° apart (i.e. one is inverted). If the amplitudes are the same, the output is zero. As in really zero - nothing at all. This applies whether you have 1V or 1kV sinewaves - they cancel perfectly.
If one output is shifted by a mere 1° (181 or 179° phase shift between the two), with 1V inputs, the sum is 8.72mV, or -41dB. You can calculate this for any phase angle with the following formula ...
Output = VIN × sin( θ ) / 2 Where VIN is input voltage and θ is the phase angle between the two voltages
Unfortunately, it's not at all difficult to accumulate a 1° phase difference between two seemingly similar circuits, especially at higher frequencies, and doubly so if they are cascaded (one following another). For most things it doesn't matter (and doesn't even happen between two hi-fi signal channels), but when you are trying to cancel a wide band signal it matters plenty. In similar manner, if two equal and opposite voltages (assume 1V) are summed with 10k resistors, a difference of just 10Ω (1 in 1,000 or 0.1%) will cause an output of 500µV at the summing point. In general, expecting better than 60dB of CMRR is unrealistic unless you are willing to use 0.01% tolerance components. These are not readily available, and are expensive.
Another fairly popular circuit is known by a few names, such as 'earth/ ground cancelling output' or 'ground compensated output'. It does provide a balanced output, but it is impedance balance only, and there is no signal on the second ('cold') line.

Figure 3 - Ground Compensated Circuit
It should be obvious that the circuit shown has no output common mode rejection as such. What it does instead is to use the noise signal to cancel any noise that would otherwise appear across the load. Noise will appear on both signal lines, and the 'cold' (-Out) lead couples that back into the opamp in such a way as to cause the noise signal be cancelled. Cancellation can never be total of course, due to normal opamp limitations, but a significant part of the 'ground noise' can be effectively removed. This arrangement works whether the remote load is balanced or unbalanced.
The next circuit shows how two opamps can be forced to operate in an almost identical manner, so any inherent phase shift difference is minimised. Although they seem to be operating more-or-less identically, in reality that's not the case. U1B is operated as a unity gain inverter, so has (almost) zero common mode signal at its input terminals, as both remain at (nominally) zero volts. However, U1A does have a common mode input voltage, namely half the input voltage. This means that the two are not identical, but they are much closer than obtained by the Figure 1 circuit.

Figure 4 - Optimised Balanced Output Driver Circuit
You should recognise the circuit based around U1A - it's the standard single opamp balanced input circuit (but it is drawn differently). The circuit has a gain of two, so the input voltage is divided by two at the non-inverting opamp input, ensuring that the output signal is the same amplitude (and phase) as the input signal. You can use the complete balanced circuitry for both opamps if you wish. Then both are identical, but one is driven via the +ve input and the other via the -ve input. However that provides no benefit, and only increases noise and component count.
The CMRR at the output is greater than 96dB up to 3.7kHz, and is still better than 87dB at 20kHz. This is a fairly dramatic improvement over the Figure 1 design, but it adds 4 more resistors - all of which must be close tolerance. In most cases it's not necessary, but if you are after the best possible result it works well. The input impedance is 6.67k with the values shown, and it expects to be driven from a low impedance (such as the output from an opamp). Noise performance can be improved by using lower value resistors throughout, but at 'line' levels it's unlikely to cause a problem.

Figure 5 - CMRR Of Output Voltage
This is the CMRR response, plotted again from 10Hz to 100kHz. The difference is immediately obvious. While the (in reality unlikely) maximum CMRR of better than 100dB at low frequencies is limited to a 'mere' 97dB below 1kHz, it remains at that level, where the previous circuit was rising steadily from a few Hertz. Note that the vertical scale is compressed, and even at 100kHz the CMRR is greater than 70dB. Whether you can achieve results this good in a real circuit is doubtful, but the potential clearly exists. Substituting different opamp models in the simulation does change it a little (some are better, others worse), but the results generally follow the trend shown. Regardless of opamp, it will always outperform the basic circuit (Fig. 1).
In all circuits that follow, CMRR is measured by tying the two inputs together and applying a 1V signal to the two inputs at the same time. An ideal circuit would mean that the output would be zero, implying infinite common mode rejection. As should be apparent by now, the ideal opamp does not exist - and that includes expensive discrete designs that are often no better than decent integrated circuit types.
Make sure that you have a look at the article on Instrumentation Amplifiers (INAs), because that describes them in greater detail than you'll find here. In reality, most of the balanced receiver circuits shown below are also considered to be 'INAs', even when they are quite obviously not the full implementation of the 'true' INA circuitry. A comparatively new (at the time of writing) device is the INA1650 [ 9 ], which includes just about everything that is needed for a balanced input. It's claimed in the datasheet that CMRR is better than 70dB to well over 100kHz. Like so many of the latest devices, it's only available in a surface-mount package.
The standard single opamp balanced input circuit generally gets a bad rap for performance, largely because it has unequal input impedances on each of its inputs. However, this is largely a distraction, because much of the time it doesn't matter. If it's fed from a true balanced source (i.e. not earth/ ground referenced) it's immaterial. The source sees the total impedance, and the common mode performance is usually much better than most people give it credit for.
However, the unequal impedances may cause problems in some installations, in particular where the source signal is earth referenced (i.e. a symmetrical signal about zero volts, with an actual or inferred earthed centre tap). Both balanced driver circuits shown above have just that - there is no output 'earth' as such, but both signals are directly referred to the zero volt line (earth) because they are driven by opamps. While it is possible to create a 'pseudo-floating' output using opamps, the circuit relies on some positive feedback and it may become unstable under some conditions. It's the closest electronic equivalent to a transformer, but it's still not as good because there is no galvanic isolation.
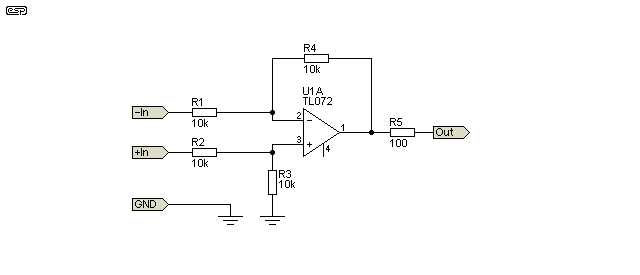
Figure 6 - 'Conventional' Balanced Input Circuit
The traditional balanced input stage is shown above. The input impedance of each individual input depends on the source - it's not a fixed value, and is different for common mode and differential inputs. This has convinced many people that it can't work properly, but that is not true at all. It is a compromise, but it's not as bad as it appears at first look. With a fully floating input (a microphone capsule for example), you'll actually measure very different voltage at each input pin. With a 1V source, at the non-inverting input you'll measure 1V, and almost nothing on the inverting input.
While this is somewhat confronting, it actually doesn't matter. You still get the output voltage you expect (1V), and common mode noise is rejected just as effectively as any other arrangement. With a common mode signal, the current into each input is identical, and therefore, the common mode impedance must also be identical. Signal balance is not a requirement for a balanced line (although many people expect it). The thing that makes a balanced line balanced is its common mode impedance - if the impedance is equal, then the line is truly balanced.
Look closely at the Figure 6 circuit, and assume that the output of U1A is zero (which it will be for a common mode signal). We shall ignore any output DC offset, as well as the output impedance of the opamp for this exercise. With the common mode signal (i.e. applied to both inputs simultaneously), each input 'sees' a voltage divider and two 10k resistors in series. The input impedance for each input is therefore 20k (10k + 10k, and ignoring the input impedance of the opamp), so the impedances are perfectly balanced. They are different for a differential mode signal, but that doesn't matter!
For example, if a 1V common mode signal is applied to each input, the current into each is 50µA - an impedance of 20k. If the common mode signal is applied to each input via two external impedances (as may be the case with a shielded mic cable), the input current remains identical. For example, an external 10k on each input reduces the current to 33.33µA, so the impedances are quite obviously the same, provided the impedance of the source (including cable) is also balanced.
Adding input filters to remove signals much above 20kHz means that it's easy enough to ensure at least 90dB of CMRR up to any sensible frequency. A suitable filter might be 1k in series with each input, with 3.3nF to ground. The two 3.3nF caps must be very carefully matched or the CMRR will be seriously degraded. This arrangement is shown next.
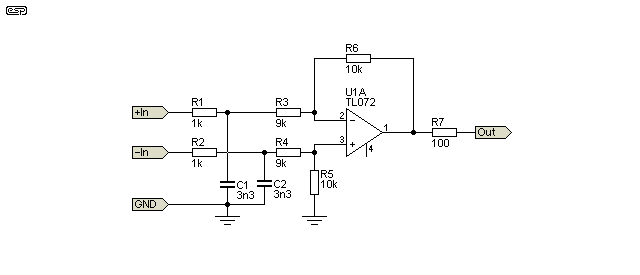
Figure 7 - Conventional Balanced Input Circuit With Input Filters
The filters use R1/2 and C1/2 to create a low pass filter, tuned to 48kHz. The source must be low impedance, or the filter frequencies will be reduced, potentially leading to a loss of high frequencies. R3 and R4 have been reduced from the nominal 10k to 9k to ensure that the gain is maintained at unity, but in reality you can easily use 10k instead and accept the small gain reduction.
The two caps must be exact - the absolute value isn't especially important, but the balance between them is critical if a high CMRR is expected. There are some tricks that can be used to make the filters less critical [ 2 ]. There are several integrated versions of the basic balanced input circuit that, while basically complete within the IC itself, offer no real advantage other than a smaller PCB area.
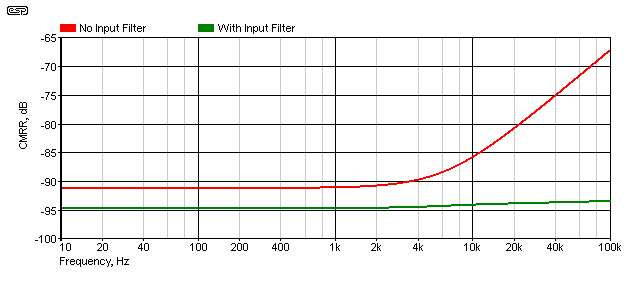
Figure 8 - Input CMRR Of Figures 6 And 7 Circuits
It's hard to argue that the result shown in Figure 8 is poor, because it isn't. The result is primarily determined by the opamp, but even with a lowly TL072 it's a good result, with a CMRR of better than 90dB up to just under 10kHz. When the input filters are added, the CMRR is better than 90dB up to any sensible frequency.

Figure 9 - Balanced Input Stage (Project 87A)
The circuit shown above is the same as that used for Project 87A. It has the advantage that the input impedance can be as high as you like, based only on the opamps' input current (which is negligible for FET input types). Common mode rejection is acceptable generally, but the optional 'phase lead' network (which must be adjusted to suit the opamp being used) improves matters. Without it, the CMRR is still 30dB at 20kHz, but the phase network can improve that to about 58dB at 20kHz. Use of input filters as shown in Figure 6 improves CMRR further if high frequency common mode noise is an issue.
R7 can be installed to increase gain. If R7 is omitted, the circuit has a gain of 6dB (x2), and it cannot be reduced without adding voltage dividers to the inputs. If R7 is 10k, the circuit has a gain of 12dB (x4). Reducing the value of R7 increases the gain further (e.g. 1k gives a gain of 26.8dB (x22), but CMRR is reduced as the gain is increased. CMRR is reduced by (roughly) the same amount as the gain is increased, so 20dB gain means 20dB worse CMRR. If R7 is used, the phase lead circuit (if used) must be adjusted to compensate.

Figure 10 - 'Super Balanced' Input Stage [ 1, 10 ]
This circuit has been described by Douglas Self (he calls it the 'Superbal'), and it was invented by Ted Fletcher [ 10 ]. It ensures that the impedance at both inputs is the same as the input resistors (10k in this case). For the most part this doesn't give quite a much benefit as you might imagine, but the equal impedances certainly help to ensure that the CMRR isn't compromised by the unequal load on each signal line. The CMRR is very slightly better than the Figure 6 circuit at low frequencies, but by 20kHz there's no difference. One thing that is not mentioned is that the output voltage is half that of the Figure 6 circuit (-6dB) because of the feedback via U1B. This is of no account for line inputs operating at +4dBu or so. Total input impedance is 20k as shown, with each input having an impedance of 10k.
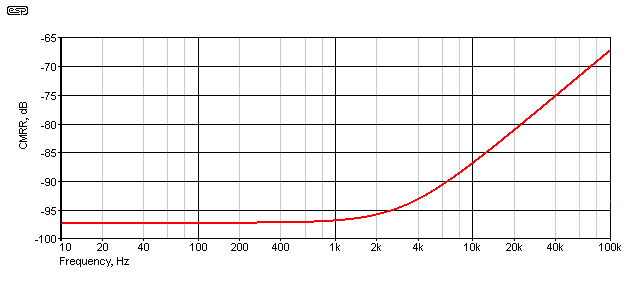
Figure 11 - 'Super Balanced' Input Stage CMRR
As before, adding input filters will improve the CMRR at high frequencies. As is obvious, the CMRR is very similar to the 'conventional' version, with the only real difference being that both inputs now have the same impedance. If you happen to think that's important, then it's well worth using, but mostly it doesn't matter a great deal.
FDAs are a convenient way to make a circuit that can accept balanced or single-ended inputs, and provide balanced or single-ended outputs. This means an FDA can convert unbalanced to balanced, balanced to unbalanced, or buffer a balanced connection (balanced in/ out). Some are designed for low voltage operation (e.g. 5V) which is useful for interfacing with ADCs (analogue to digital converters) or balanced DACs (digital to analogue converters), but their input and output voltages are too limited for professional audio or anywhere that a decent signal level is expected.
One example of a 'full supply' (up to ±16.5V maximum) is the OPA1632 - but it is only available in SMD packages. Many others are also unavailable in standard DIP packages, making them less suitable for DIY projects. Unlike an opamp, an FDA has two inputs (inverting and non-inverting) and two outputs (also inverting and non-inverting), and two separate feedback paths are used. The same caveats regarding resistor tolerances apply with the FDA, so if maximum CMRR is expected, close tolerance parts are essential. Most also provide the ability to have DC offset correction, or to create a fixed DC offset to match the requirements of ADCs.
While these devices appear to be the answer to all your balanced/ unbalanced conversion woes, they have similar limitations to maximum CMRR at high frequencies as you find with opamp circuits. Some are designed to handle video, so have a much wider bandwidth than most opamps (up to 200MHz for unity gain), but sadly there are usually no graphs that show input and output CMRR with respect to frequency. In use, I doubt that any will be found wanting, other than those using 5V supplies. These are not suitable for general purpose audio line-in or line-out applications.
The equivalent circuit is fairly convoluted, and while I show the (claimed) equivalent circuit for the OPA1632 below, I do not propose to go into great detail by way of explanations. The equivalent circuit is 'functional', in that it shows the internal functionality, but the reality is somewhat different. The circuit shown has been simulated and it works, but the output voltage is limited to around ± 3.7V rather than ±12V (with 15V supplies) that you'd normally expect. Note that this is for the simulation - the 'real' OPA1632 extends to at least ±12V with 15V supplies, depending on the load impedance.

Figure 12 - OPA1632 Functional Equivalent Circuit [ 6 ]
Essentially, the circuit is similar to that for an opamp. The difference is that rather than providing a single output, there are two, with one for each input. When two independent feedback paths are added, it allows very flexible input and output options. The VOCM input allows the designer to set a specific DC common mode voltage where this is needed. If not used (and assuming dual supply voltages), the common mode voltage will be set to zero by grounding the VOCM input.

Figure 13 - General Usage Of FDA (OPA1632 Pinouts Shown, PSU Pins Not Included)
An unbalanced input can be applied to either input pin, and the other is grounded. A balanced input is applied to both input pins, and no ground is needed, although providing a DC path to ground is required. If an unbalanced output is needed, the output can be taken from either output pin, and the unused one is left floating. This means that the one IC can be used for balanced in to unbalanced out, unbalanced in to balanced out, or balanced in to balanced out. Despite what you might think, the output is (or is not) true unity gain, depending on your expectations. This is despite the equal value input and feedback resistors. As shown, the gain is 'unity' only in that a 1V unbalanced input provides a 1V balanced output, and a 1V balanced input gives a 1V balanced output. The actual voltage on the each output pin is 500mV, and being 180° out of phase, this is 1V.
The gain is changed in the same way as with an inverting opamp circuit. If the feedback resistors (R3 and R4) are made twice the value of the input resistors (R1 and R2), the gain is two. Input impedance at each input is 10k as shown. If a higher input impedance is needed, you'll have to add input buffers or increase the value of the input and feedback resistors, which will increase resistor thermal noise. The two 100 ohm resistors at the outputs serve the same purpose as they do with an opamp circuit, and isolate the output pins from capacitive or resonant loads (such as cables).
In the case of FDAs, there is no substitute for the datasheet and/ or application notes. I could ramble on for many paragraphs trying to explain the things you can (and can't) do with an FDA, but most of it would have to come from the datasheet anyway. It may take a while to understand the many options that may be available, but it's worth persevering if you need a single-chip solution to balanced and unbalanced conversions. Also, bear in mind that FDAs are usually fairly costly, and it will usually be cheaper to use opamps for 'line level' applications.
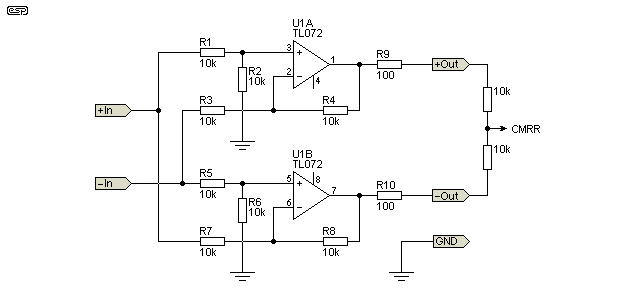
Figure 14 - Fully Differential Amplifier Using Opamps
You can create an FDA using a pair of opamps as shown above. Quite a few resistors are needed, and as before they must all be close tolerance. The circuit is simply a pair of differential input amps, with the inputs cross-coupled. The input can be applied to either the '+In' or '-In' terminals, with the unused terminal grounded. A balanced input can be applied between the two inputs in exactly the same way as an integrated FDA. No additional ground reference is needed because of R2 and R6, so a balanced source can be fully floating.
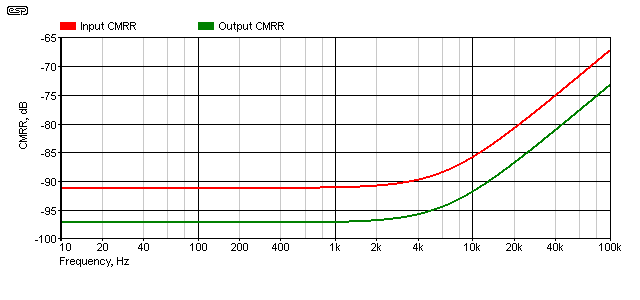
Figure 15 - Output/ Input CMRR Of FDA Using Opamps (Exact Values)
With the values shown, the input impedance is 6.67k to each input (13.34k for a floating balanced input), and the circuit has unity gain at each output (so it has an overall gain of x2). Simulated output CMRR is better than 80dB up to 40kHz, but of course that's using resistors of exact values. Input CMRR is better than 70dB up to 40kHz (again with exact values). You will never achieve the best case performance even with 0.1% resistors, but input and output CMRR can be expected to be better than 40dB with 1% resistors throughout. (A simulated 50-step Monte Carlo analysis with all 10k resistors varied by ±1% shows worst case input and output CMRR to be 40dB.)
This is a versatile circuit, and will work well for either balanced to unbalanced or unbalanced to balanced conversion. However, it does have a relatively low input impedance because the source has to drive the inputs of both opamps. In most respects, it should work at least as well as an integrated FDA, but of course it doesn't have provision for DC offset. While this could be added, there's no point for a normal line driver or receiver.
While a transformer may (theoretically) provide infinite CMRR for inputs or outputs, the reality is different. By necessity, transformers have at least two windings, which are coupled by magnetic induction. However, there is also some capacitive coupling between the windings, and this degrades the common mode rejection, especially at high frequencies. Transformers are also only usable over a relatively limited frequency range, with perhaps 3 decades (say from 20Hz to 20kHz) being readily achievable (with a little to spare for a well designed component). An electrostatic screen between primary and secondary helps minimise capacitive coupling.
If exceptional common mode performance is needed the transformer almost certainly needs to be driven by a balanced driver, (or followed by a balanced receiver for an input circuit). If there is significant capacitive coupling between the windings, using balanced drivers or receivers won't help a great deal - if at all.
One thing a transformer (even a cheap one) does provide is galvanic isolation. This means that there is no ohmic connection between the windings, and this isolation barrier may be used for electrical safety and/or to isolate sensitive circuitry from a hostile external environment. Naturally the transformer must be rated for the degree of isolation required, so using a cheap 1:1 10k transformer (around $2 to $3 on-line) for 230V mains isolation is not an option.
Unfortunately, decent transformers are expensive, and this limits their usage in many cases. It's also unfortunate that CMRR is usually very good (even for cheap types) at low frequencies, but falls at high frequencies due to inter-winding capacitance. This is also where opamp circuits are limited, so the benefits might not be a great as hoped for. Unfortunately, it's very difficult (mainly time consuming) to build a simulation model for a real transformer, so I measured one that I have to hand. It's nothing special (quite the reverse in fact), and is nominally 10k 1:1 ratio. Inductance measured 200mH, but is actually higher because inductance meters usually don't work well with transformers. Winding resistance is 130 ohms, and there's 3.6nF capacitance between primary and secondary.
It's the capacitance that ruins everything. CMRR at 100Hz is excellent (as expected), but at 10kHz it's only 30dB, falling to 21dB at 30kHz. Adding a balanced opamp stage at the transformer's secondary is not as helpful as you would hope, because the opamp's CMRR is poor at high frequencies too. The only way to get good results at high frequencies is to use a transformer with an electrostatic shield between the windings.
In general, transformers should be driven from the lowest practicable impedance. There are advantages to using negative impedance [ 8 ], but this isn't always practical or even possible. The primary winding resistance means that even a transformer driven from a zero ohm source still has a defined source impedance - the primary winding resistance). Negative impedance can be used to cancel most of the winding resistance, allowing closer to zero ohm source impedance.
It's no accident that when valve circuitry was the standard, balanced inputs and outputs were transformer based. Valves simply don't have the gain to allow much feedback, and the matching between them isn't good enough to rely on without trimming (which may be needed several times during the life of a set of valves). Their output impedance is too high to drive a nominal 600 ohm line without a transformer, and they are best avoided in this role.
Of course, it is possible to use valves, but the performance will never even approach that you can get with ICs - dedicated or otherwise. Even a 'solid-state' discrete design will be vastly superior to any attempt at an 'equivalent' valve circuit without a transformer. The cost will also be a great deal higher and power consumption much greater. There are no sensible reasons to even try to use valves for direct-coupled balanced drivers or receivers.
We often expect perfection (or something close) with electronic circuits, but as shown this is an impossible dream. What we can achieve is results that are 'good enough', which doesn't mean they are inadequate - it means that even if they were significantly better we would (probably) not hear any difference. Many of the common circuits have been in use for years, and there's no evidence that the 'limitations' cause problems in a well set up system.
Ultimately, circuit design (as with most engineering) is an exercise in compromise. This can even be classified as an 'art form', because the designer has to trade many limitations with many others. The 'art' comes into play to decide those parameters that have the least effect on the desired outcome, all the while ensuring that the circuit remains within budget and is practical. Even ignoring the budgetary constraints for commercial products doesn't mean that the outcome is 'better' than it would be if all parts used were of the highest specification possible. If the end-user can't hear a difference (in a double-blind test of course), then the extra cost and complexity is wasted.
In particular, it's pointless designing any circuit that requires parts that are difficult to obtain (especially obsolete components). In essence, using 'unobtianium' parts is equivalent to basing a design on an IC that hasn't been invented yet, and probably never will be. Compromise is essential for both manufacturers and hobbyists, or the project is doomed to failure because no-one can get the part(s) for it.
If you are happy to use 0.1% resistors (they do tend to be rather expensive - most are over AU$1 each), then your overall CMRR can be improved further. For those with an unlimited budget (there aren't too many), you can get 0.01% tolerance, but for those you pay very dearly indeed (typically over AU$20.00 each!). Of course you can select resistors from the 1% range, but thermal stability may not be as good as true precision components.
Where noise (hum loops in particular) is especially troublesome, often a transformer is the only option. One positive is that only one end (either the line driver or receiver) needs a transformer, and the other end can be 'electronically balanced' using one of the circuits shown here or in the other referenced pages. This arrangement maintains a balanced connection, and includes the galvanic isolation of the transformer. If noise persists, it's far better to find out where it's coming from and fix the source of the noise, rather than trying to keep it out of cables, preamps, etc.
A transformer is the only option if there is a significant voltage differential between the source and destination circuits. This may be due to earth (ground) current, different mains circuits wending their way back to the switchboard, and possibly with the mains being derived from different phases of a three-phase installation. Some circuits require extreme isolation (medical instruments being a case in point), and even a 'conventional' audio transformer will probably be incapable of providing the safety rating (and pass all relevant standards) required. This is another topic altogether of course.
Dedicated ICs can provide very good results, but most of the time they aren't necessary. With signal levels of around 1V, even a troublesome system may only have a few millivolts of common mode noise. If this can be reduced by 40dB (generally fairly easy to achieve even with unmatched 1% resistors), then the noise voltage is reduced 100-fold. Even 10mV of noise is reduced to 100µV, and the 40dB signal to noise ratio (1V signal, 10mV noise) is increased to 80dB. By means of careful circuit layout and well made (and sensibly run) cables, background noise can be all but eliminated.
There isn't always a choice of course. Some systems are used for outside broadcasts and similar, where the environment can be particularly hostile. When this is the case you may have no alternative to a transformer. Not only does a quality part provide good common mode noise reduction, but the galvanic isolation protects the electronics from the evils of the outside world. It's rare that transformers are needed in a domestic installation, but if all else fails this may be your only solution to intractable noise problems.
1 Balanced Interfaces - Douglas Self
2 Balanced Interfaces - Bill Whitlock
3 Balanced Line Driver with Floating Output - Uwe Beis, Rod Elliott
4 Projects 87A and 87B - Balanced Line Drivers & Receivers
5 Instrumentation Amplifiers Vs. Opamps - Rod Elliott
6 OPA1632 FDA Datasheet - Texas Instruments
7 Fully Differential Amplifiers - Texas Instruments
8 Negative Impedance - What It Is, What It Does, And How It Can Be Useful
9 INA1650 - Texas Instruments
10 Ted Fletcher's Website - (Inventor of the 'Superbal' circuit)
 Main Index
Main Index
 Articles Index
Articles Index