

|
| Elliott Sound Products | Project 17 |
I have to say from the outset that I do not agree with the use of weighting filters, since they are not (despite the claims, standards and legislation to the contrary) an accurate representation of human hearing, nor do they predict the potential for annoyance to people other than by accident.
In fact, the standard A-weighting curve is accurate at only one SPL (Sound Pressure Level), assuming that the listener has "British Standard" Ears. I have no idea what SPL this filter is meant to be accurate at, and I doubt that anyone else does either. Based on the "Equal Loudness Curve" (see below), the closest match is at or near 30dB SPL - an unrealistically low noise level by today's standards. At a rough guess I would suggest the A-weighting curve may have some small relevance somewhere around 40dB SPL (unweighted!) and below. However ...
Any noise measured using A-weighting must be free from tonality or rhythm, and will be a broad bandwidth random signal. For the vast majority of real-world measurements that do not fulfil these criteria, A-weighted noise level measurements give a completely unrealistic reading that does not reflect audibility or annoyance value of parts of the sound ... especially very low frequency signals (modulated or otherwise) and/or any rhythmic sound.
When the police measure the noise from a car exhaust or a party, they happily use A-weighting - it's in the legislation - that has to be scary! Politicians and bureaucrats thinking that they know about SPL? Next thing they will tell us that they understand fiscal policy. But I digress ...
The purpose is supposedly to account for the fact that human hearing is less sensitive at low and high frequencies than in the upper midrange, and that this variation is dependent upon the sound intensity (SPL). The Fletcher-Munson curve (as it is commonly known, and reproduced below) shows the variation, and it is clear that any loss of sensitivity is highly dependent upon the actual SPL. The idea that a single filter can represent the true subjective annoyance potential at all levels is clearly seriously wrong, but it is a standard nonetheless, and its use is mandated in most countries.
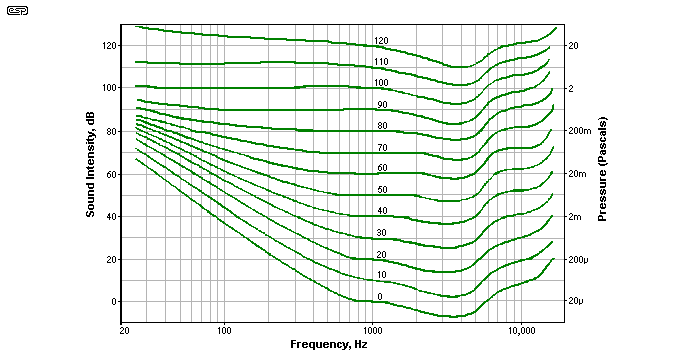
Figure 1 - Equal Loudness Curves (After Fletcher and Munson)
The premise behind all this is that as the SPL is reduced, our ability to detect low or high frequency noise is reduced, so measurements should reflect this phenomenon. While it is undeniable that the chart above represents reality in terms of human hearing [1], I remain unconvinced that A-weighting is a valid test methodology unless the absolute sound intensity is specified. In addition, it only works with wide band noise. If a sound has a rhythm or tonality, you cannot use A-weighting to measure the likely 'annoyance value' and the meter will badly underestimate the audibility of the noise.
Ok, I agree that there just might be some validity hiding in there somewhere for thermal noise measurements of amplifiers and the like, but again, any tonality changes everything. Just because the meter tells me that I should not be able to hear the harmonics of the 50/60Hz mains, does not mean that I cannot. There are some sounds that seem (at a casual glance) to defy all measurement standards, and remain audible (albeit at very low level) despite all the 'evidence' that this should not be so. As with all such things, experience and practical application are more important than the absolute indication on a meter.
A piece of equipment that is essentially 'noise-free' for all intents and purposes is in reality a waste of time, since the ambient noise level in most urban or suburban areas is likely to be far higher than the residual noise of most audio equipment. How useless is 100dB signal to noise ratio for a car hi-fi system, for example? Even the most expensive luxury cars generate far more noise than any tuner/cassette/CD system (and this is apart from all the other external noise generated by other vehicles on the road).
It is worth noting that the Fletcher/ Munson curves were devised in 1933, with a test group that apparently consisted of only about 12 people. Equipment of the day was seriously lacking by today's standards, response was plotted between 25Hz and 16kHz (in 1933 even that was quite a feat!), yet the above curve is considered to be gospel throughout the industry. I'm not disputing that the general trends are accurate (there would have been changes if errors were found), but I am astonished that test data from so long ago have managed to stand the test of time.
Since it is unlikely that I shall be able to convince the entire industry that it is using flawed reasoning, I shall describe an A-weighting filter so that we can at least make some meaningful comparisons with other systems where this has been used. Note that A-weighting is generally applied only to noise measurements, so might have some validity in this respect ... as long as the noise we are measuring is of very low amplitude, has a broad frequency spectrum, and contains no tonality or rhythm - the neighbour's party and most other urban noise sources are unlikely to fit this mould, but will be measured with A-weighting anyway - oh dear - so much for getting some sleep! (And yet again I digress ... )
The curve of the described filter is shown in Figure 2, and it can be seen that it is essentially a tailored bandpass filter, having a defined rolloff above and below the centre frequency. The reference point is at 1kHz, where the gain is 0dB. The filter response is supposed to be the inverse of one of the curves of the equal loudness graph shown in Figure 1 - it is a little hard to tell which one, but this is a standard, so we shall leave it at that. For anyone who wishes to be able to reverse the filter, Project 130 describes an inverse A-weighting filter that is within 1.5dB of being flat over the range of 20Hz to 20kHz (-3dB at 11.3Hz and 30kHz).
As can be seen from Figure 3, the circuit is very simple, but even with this frequency response it is not particularly hard to calibrate. Regardless of what may be claimed though, I do not accept for an instant that it really does account for our perception of real-life noise levels. It is really a laboratory curiosity and as such might be useful for research but little else.
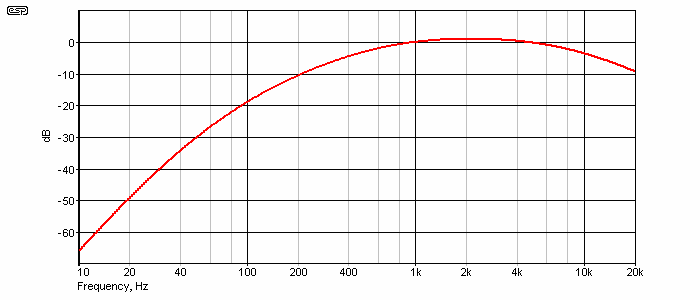
Figure 2 - Frequency Response of the A-Weighting Filter
The filter itself is passive, and the opamps are there only to buffer the input and output, and to adjust the gain so there is some correlation with reality (however slight). Note that the input impedance is quite low, and the output impedance is high, so the unit should be well shielded to prevent noise pickup from the outside world.
As always, I suggest the use of 1% metal film resistors, and the capacitors should be measured and selected, or close tolerance types used. If 'ordinary' capacitors are used, their tolerance will adversely affect the accuracy, but for normal use (i.e. non-certified laboratory), it should be close enough even if 10% caps are used. After all, the noise level of any semiconductor amp (compared to another) is likely to be somewhat variable anyway, and extreme precision is not normally warranted. Remember that mains hum may not register when A-Weighting is used, but will often be audible regardless.
The circuit can be operated from a pair of 9 Volt batteries, or a regulated supply of up to ±15V. There is no need to use premium opamps unless extremely low noise levels are to be measured, and even then are not needed if there is a gain stage at the front end.
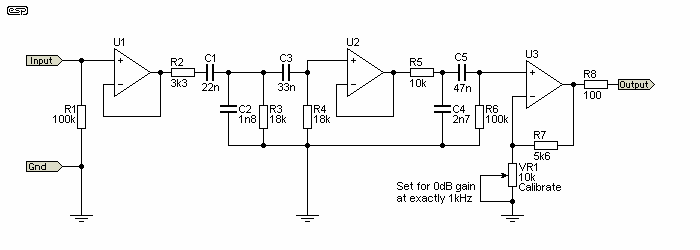
Figure 3 - The A-Weighting Filter Schematic (2023 Version)
I will leave it up to the reader to decide on the opamps - Most general purpose opamps should be ok for most applications. No opamp pinouts have been included, these are available on any manufacturers' data sheet if you don't know them. The requirement for three opamps is a nuisance, but it makes the filter design much easier to drive, as very low impedances can be avoided. The previously published circuit (see Fig. 4) has an input impedance of less than 1kΩ over much of the frequency range, and that's a difficult load for an opamp to drive.
All resistors and capacitors are from the E12 series, and caps should be selected to be within 1%. Resistors will be 1% metal film types. The response is well within the tolerance window for an A-Weighting filter if al parts are within 1%. There are four points that should be accurate - 20Hz (-50dB), 1kHz (0dB), 3kHz (+1dB) and 20kHz (-10dB). The filter described is within 1dB at all of these frequencies.
Basic calibration is not hard - the overall gain at 3kHz is 1dB, so if the input is set to 1V RMS, the output at 3kHz should be 1.12V. Alternatively, at 1kHz, the gain (or insertion loss) should be 0dB - I would suggest that it is checked at both frequencies if possible, and if necessary, average the error between the two frequencies.
Use the 10k trimpot to adjust the level (you need to be accurate with your measurements if true A-weighting is to be obtained). Note that the trimpot should be a quality multi-turn type to enable accurate setting and long-term stability. Alternatively the trimpot may be replaced with a 5.6k resistor, and accuracy will be quite acceptable for most applications (the error is less than 0.2dB). The gain required from U3 is 1.95 (5.8dB) to get the level correct (0dB gain) at 1kHz.
So, there you have it. This project will enable you to make 'industry standard' measurements of amplifier noise levels, it is up to you to decide which particular standard you want to make comparisons against. Life would be so much easier if all noise measurements were made 'flat' - with no filters of any kind, but this is not to be. A sensible filter is what's commonly known as C-weighting for noise measurements, with noise below 20Hz and above ~15kHz being filtered out. Much as many people would like to see the standards changed (including the World Health Organisation), I fear that it won't happen unless enough people point out that the present A-weighted measurements are largely meaningless because they are misused due to an almost complete lack of understanding.
If anyone is demented enough to want to do so, you can calculate the A-Weighted level at any frequency. I must confess that I have done just that, but only to prove to myself that the formula works (promise  ). The formulae can be found in several websites, including Wikipedia. I've only shown that for A-Weighting, but there's another for C-Weighting and even B-Weighting (no longer used). The formula (despite its complexity) is approximate. An offset must be applied to get the true value and to determine dBA.
). The formulae can be found in several websites, including Wikipedia. I've only shown that for A-Weighting, but there's another for C-Weighting and even B-Weighting (no longer used). The formula (despite its complexity) is approximate. An offset must be applied to get the true value and to determine dBA.
Ra(f ) = 12200 2 × f 4 / ( f 2 + 20.6 2 ) × ( f 2 + 12200 2 ) √(( f 2 + 107.7 2 ) * ( f 2 + 737.9 2 )
dBA(f ) = 2 + 20 × log( Ra(f ) )
Predictably, f is the frequency. These formulae only work for single frequencies, and they can't be used for frequency bands. Note that frequency weighting responses are defined in the standards by tables with tolerance limits, and not by the formulae shown.
For example, if you run the calculation for 20Hz, you get 31.33dB, and 81.75dB at 1kHz. The difference between these is 50.4dB (near enough), and if you look at the graph in Fig. 2 you'll see that 20Hz is ~50dB below the 1kHz level. Accuracy at 10kHz and above is suspect.
In fact, it is quite easy to prove that A-Weighted measurements at any meaningful level are pointless. You need a speaker with good response to at least 40Hz, and a graphic equaliser that can provide about 10dB boost at 40Hz, plus either music or a pink noise source. Set up the equipment, and play the signal at about 74dB (unweighted). Prove that the meter (set for C or Z-Weighting) shows an increase when the 40Hz component is boosted, and that you can hear the difference (it should be very obvious).
Now, set the meter to A-Weighting, and repeat the test. According to the meter you cannot hear the difference, yet perversely, you find that it is just as audible as when the meter was set for C-Weighting! But how can this be? Everyone knows that you can't hear such a low frequency - just look at the Fletcher/ Munson curves above! Read the meter - it tells you that you can't hear the change. Strangely, you hear it anyway, as will anyone who comes along to find out what you are up to. At 40Hz, the signal is attenuated by 34dB, so its influence on the total level is greatly diminished.
This simple experiment should be mandatory for anyone who uses a sound level meter, and should be forced upon all legislators and standards writers. The test must be continued until the victim test subject freely admits that they can hear the difference, and the expensive meter they are clutching is therefore wrong, and should not be used for measurement of noise until they learn how to switch off weighting filters (and use their ears). Consider that low frequencies can travel a long way without significant attenuation due to the air and buildings/ vegetation, so not only is the measurement wrong, it's even worse for people some distance from the noise source.
Just in case you missed my point here ... A-Weighting is bollocks. It doesn't work, and is used by industry because it doesn't work, thereby giving them far more leeway than should be the case. I have spoken with many, many people involved in professional noise measurement, and the sensible ones (i.e. those not employed by an industry that gets noise complaints) all freely admit that A-Weighting is flawed, and is rarely used appropriately. Someone, somewhere obviously thought it was a good idea, but how it managed to become the default measurement method for all published standards remains a mystery. It's apparent that little or no 'real world' testing was ever done.
I jokingly said to some people I worked with some time ago that I could imagine a 'consultant', clutching his meter, hearing low frequency noise that obviously could not be ignored, but still pointing to his meter and saying "No, no, it's perfectly fine - look at the meter."
Unfortunately, I was advised that this is no joke - they had seen this exact scenario with their own eyes. I kid you not.
I'm not alone in disputing the usefulness (or otherwise) of A-Weighting, but those who write the standards aren't listening to anyone who argues against the way sound is measured to determine its annoyance value. This is a worldwide problem, and the serious errors made by using A-Weighting to measure everything are simply ignored. It's a shame, once something is legislated, it's in the political arena, and I'd expect that I could count the number of politicians who truly understand any of this on the fingers of one hand.
The filter as originally shown was a little off at 2.7kHz relative to 1kHz (it should be 1.3dB higher at the higher frequency), and this has been corrected. The version shown should be accurate to within about 0.1dB.
It was pointed out (May 2000) that the curve of the original filter shown was not a very good fit to more modern measurement sets, and a small modification will cure this. The low frequency response of the original was not quite what it should be, and at high frequencies the rolloff was too slow. The circuit now shows the final version which is more accurate than the original.
Modification to original circuit contributed by ...
Jürgen Fehringer
Elektronikentwicklung
AKG Acoustics GmbH
My thanks for this useful update.

Figure 4 - A-Weighting Filter Schematic (August 2002 Version)
The circuit shown above is the original from the 2002 update. It works perfectly, but the low input impedance of the filter makes it hard to drive (< 2kΩ Zin above 600Hz). The response is slightly different from the new version, but both are within the tolerance 'window' [2]. High frequency response is slightly closer to the required curve if C4 is increased to 2.2kΩ.
1 Fletcher and Munson, Journal of the Acoustic Society of America - Vol.4, No. 2, 1933
2 Audio Weighting Filters (Mathworks)
3 A-weighting in detail (Lindos, UK)
4 A-Weighting, Sound Level Measurements & Reality (ESP)
 Main Index
Main Index
 Projects Index
Projects Index